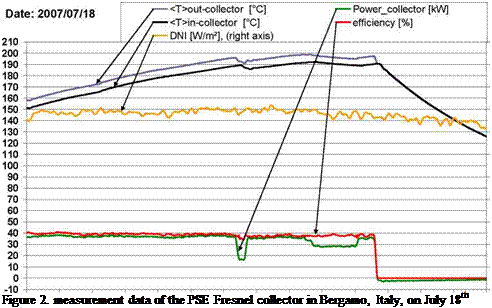
Figure 2 shows the measurement data of the Bergamo collector on 2007/07/18, a sunny summer day. During this day only 10 out of 11 mirror rows were operated. Until 14:07 the collector power stays between 35 kW and 40 kW. On this day first tests of the automatic outlet temperature control at a set-temperature of 195°C were performed. By defocusing individual mirror rows, the mirror control software limits the maximum operation temperature. At 14:07 the set-temperature was reached and the collector power was reduced by turning several lines of primary mirrors out of focus. Five out of 10 primary mirror lines were automatically turned out of focus, i. e. instead of 120 m2 only 60 m2 primary mirror area were active, whereupon the collector power dropped to approx. 50% (green line in Figure 2). The collector efficiency refers to the active primary mirror area and therefore stays on the same level (red line in Figure 2). The outlet temperature dropped from approx. 196°C to 191°C, whereupon the inlet temperature dropped with a time delay from approx. 189°C to 186°C as soon as the temperature drop reached the collector inlet.

Shortly later these five primary mirror rows were brought back into focus and subsequently fluid temperature and power were rising again. When half an hour later the outlet temperature reached 199°C two primary mirror lines were taken out of focus, so that the outlet temperature decreased slowly to 195°C. At 15:00 both mirror lines were brought back into focus — power and temperature rose again before the system was turned off completely at 15:10.

1000

900

800

700

600

500

400

300

200

100

0

12:30 12:45 13:00 13:15 13:30 13:45 14:00 14:15 14:30 14:45 15:00 15:15 15:30 15:45 16:00

Time (GMT +1h)

Date: 2008/07/16

1000

900

800

700

600

500

400

300

200

100

0

8:00 9:00 10:00 11:00 12:00 13:00 14:00 15:00 16:00 17:00 18:00

Time (GMT +1h)

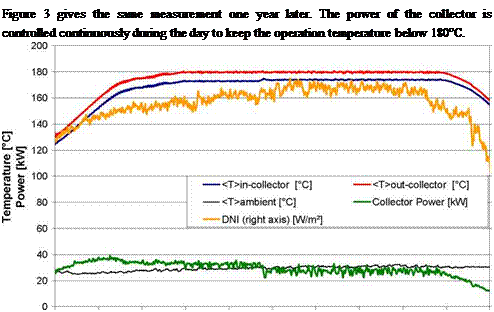
Figure 3. measurement data of the PSE Fresnel collector in Bergamo, Italy, on July 16th 2008;

The used absorber system is built of a standard sydney tube (see Fig. 4). This kind of absorber con­sists of a double walled glass pipe (1 and 2) with an evacuated annular gab. This reduces heat losses due to heat convection and heat conduction. On the outer surface of the inner glass wall (2), a selective layer is applied facing the evacuated gap, which allows the highly efficient absorption of the solar irradiation und avoids the heat losses by infrared radiation. A heat conducting system (3 to 5) is inserted over the whole length inside the inner glass pipe. It consists of a round bent heat conducting sheet of aluminium (3), which surrounds a U-bended copper pipe (4 and 5). The heat conducting sheet is attached to the inner side of the inner glass wall and transports the heat from the absorber layer to the copper pipe. The heat transfer medium streams through the U-bended copper pipe and transports the heat out of the absorber system. Inlet and outlet are located on the same side of the vacuum tube. The described kind of vacuum pipe is used in common evacuated tube collectors.

During the tests, it turned out that this kind of absorber is improper for the application in parabolic through collectors. The main reason is the temperature range, since the vacuum tube is supposed to work at temperatures between 100 °C and 150 °C at continuous operation and at a stagnation tem­perature around 200 °C, while the application of parabolic trough collectors starts at these tempera­ture ranges. Calculations have shown that at outlet fluid temperatures of 160 °C at continuous op­eration, the temperatures at the inner wall of the glass pipe already reach 210 °C.

The described effects increase because of the following reasons: the relatively high fabrication tol­erance leads to air gabs between the heat conducting sheet and the inner glass wall. The air acts like a heat insulation, which limits the transport of heat from the selective layer to the heat con­ducting sheet, with the result of an increase of temperature of the inner glass wall. For this reason the thermal losses increase and the efficiency of the system decreases.

During the measurements the glass pipe broke several times. Presumably the copper tube expanded due to the high temperatures in its length and U-turn, which resulted in a burst of the inner glass tube (see Fig. 5).

Planned optimization: The German manufacturer NARVA will develop a new absorber system with the required characteristics. A conceivable concept might be an evacuated one walled glass tube with a heat conduction system inside. The heat conduction system might consist of a U-tube combined with a bent metal sheet, which is applied with a selective layer on the surface. The inlet and outlet pipes are located on the same side of the absorber. This design is likewise the sydney tube but unlike the prototypes’ concept the U-tube does no longer implicate the danger of destroy­ing the absorber system in the new concept. It can expand inside the one-wall glass tube without colliding. Thus no critical tension is reached inside the glass.

Another opportunity would be to design a direct flow absorber with the inlet on the one end and the outlet on the other end of the absorber. Such kind of absorber would allow an easy piping of sev­eral collectors in one row as it is standard for large scale collector systems like the EuroTrough.

The system used for the solar absorbers’ testing is a laboratory scale system for domestic hot water heating (E 202, Gunt Germany). The stand image and its components are presented in Fig. 1. The commercial stand consists of a flat plate collector with the absorber plate fixed on the heating serpentine. The device was modified allowing the replacement of the absorber plate (1), while using the same serpentine during all the experiments. The solar absorber converts the simulated solar radiation, delivered by a halogen lamp (2), into heat which is transmitted to a heat transfer liquid (water). The light amount and density can be adjusted by modifying the lamp height or the solar collector inclination angle. A pump is used for water circulation through a warm water reservoir, equipped with a small heat exchanger. Adequate sensors are used for temperature (inlet, outlet and water tank), illumination and flow rate monitoring. Using a data acquisition card, the measured values are transferred to a PC for further processing. [1] [2]

Pn = б-P-cpT — T1)

Where:

— PN: is the thermal power, [W];

— cp: the water specific heat capacity, cp = 4.18 kJ/kg’K;

— Ti: the inlet temperature, [oC];

— T2: the outlet temperature, [oC];

— n: the system efficiency, [%];

— Q: the volumetric flow, [L/h];

— p: the water density, [kg/m3];

— Ac: the collector area, [m2];

— E: the light density, [W/m2];

— 0: the incidence angle, [degree].

For the measurements, the water tank and the solar circuit were filled with deionised water. The lamp was fixed at constant height (70 cm) from the collector. The collector was inclined at the angles above mentioned.

3. Results and discussions

The experimental data for the (1) and (2) absorbers are presented in Table 1, while the thermal power and efficiency vs. incidence angle are illustrated in Fig. 2 and Fig. 3.

The efficiency (n) and the thermal power (PN) for the two solar absorbers mainly depend on the absorbers type, their optical properties (solar absorptance and thermal emittance) and on the irradiance incident angle.

As Fig. 2 shows, the efficiency of the solar absorber (2), is higher compared to solar absorber (1). The black nickel, on the top of the substrate has rather similar composition for (1) and (2), being obtained using the same materials and spraying parameters, except to the deposition temperature: 350oC for (1) and 300oC for (2). Thus, the higher values of the n (and also PN) can be explained, on the one hand, by the fact that the structure Cu/CuOx has a higher thermal conductivity comparing to Al/Al2O3. In this case, the energy transferred to the flow liquid is higher. On the other hand, the optical properties (solar absorptance and thermal emittance) for the solar absorber deposited on Cu

substrate are better (Table 1), thus having higher heat gain and lower heat loss capacity. Although deposition on Cu gives better results, the Al substrate has a lower price and weight.

Generally, when 0 = 0o, the solar radiation is perpendicular on the collector surface, maximum amount of energy is reaching the panel. The heat variation produced for different incidence angles is presented in Fig. 3. The results prove that the maximum heat gain is registered for incidence angles of 10…30°. These results can be the consequence of reduced reflections and/or radiation scattering for angles higher than 0. The almost 20% increased output of the copper based collector comparing to the aluminium one, at the maximum heat gain point can also be correlated with the heat conduction/storage of the two plates.

The results presented in Fig. 2 and in Fig. 3 shows that the heat gain and the efficiency are not following a similar trend. These observations could be further used in studies of the solar-thermal conversion efficiency at low radiation amounts (e. g. for cloudy days). Also, tracking systems could be recommended to maintain the most favourable inclination angles for the solar collectors, thus to get the maximum thermal power. An efficiency increase up to 11% can be registered in this case [12].

4. Conclusion

In the present study, two types of black nickel based solar absorbers deposited on copper and aluminium substrates were tested. The absorbers were incorporated in a laboratory scale domestic hot water system. These studies were developed in the research department of the centre: Product Design for Sustainable Development, in the Transilvania University of Brasov, Romania.

The objective was to obtain two low cost solar absorbers and to test their thermal performance, in laboratory conditions. The results proved that quality absorbers can be obtained by spray pyrolysis deposition technique. The CuOx/NiOx/TiO2 absorber proved to have a better efficiency and thermal power than the Al2O3/NiO/TiO2 absorber due to better optical properties and thermal conductivity. The study showed that maximum thermal power can be achieved, in both cases, for inclination angles in the range of 10-30o.

Acknowledgements

This work was supported by the Romanian Council for Research in High Education, CEEX 277/2008 grant.

References

[1] A. B.Ceedy, (1999). Handbook of Solar System Layouts, SciPress, Lisbon.

[2] G. Katumba, L. Olumekor, A. Forbes, G. Makiwa, B. Mwakikunga, J. Lu, E. Wackelgard, Solar Energy Materials and Solar Cells, 92 (2008), 1285-1292.

[3] T. Tesfamichael, PhD thesis, Uppasla, Sweeden, ISBN 91-554-4772-4.

[4] A. Biswas, D. Bhattacharyya, H. C. Barshilia, N. Selvakumar, K. S. Rajam, Applied Surface Science 254 (2008), 1694-1699.

[5] W. E. J. Neal, Surface Technology 20 (1983), 295-299.

[6] C. E.Kennedy, Technical Report NREL/TP (2002) 520-31267.

[7] M. Voinea, C. Bogatu, G. C.Chitanu, A. Duta, Rev. Chim. (Bucuresti) 59 (2008), 659.

[8] E. Purghel, M. Voinea, L. Isac, A. Duta, Rev. Chim. (Bucuresti) 59 (2008), 469.

Performance of ICS solar water heater was compared with natural circulation type solar water heater. Natural circulation type solar water heater consist of a flat-plate collector made of copper fin having absorber area 1.9 m2 and doubled walled storage tank of 100 litres capacity. The detailed design has been described by Nahar [16]. Both the solar water heaters were filled in the morning and hot water temperatures were recorded regularly. The average hot water temperature was 57.3o C and 62.0oC at 1600 hours same day that was retained to 43.0o C and 50.4o C till next day 0800 hours when tap water temperature was 17.0o C in ICS and natural circulation type solar water heater respectively. This suggest that performance of ICS solar water heater is as good as of natural circulation type solar water heater while cost of ICS solar water heater is Rs 8000.00 where as cost of natural circulation type solar water heater is Rs. 12000.00 ( 1.0 Euro = Rs 60.00).

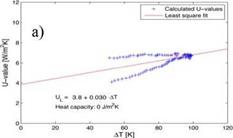
The heat loss coefficient UL is determined according to Section 2.3 for the measurements with the reference collector (not ventilated) and a collector tilt angle of 45° (data of June 10, 2006). The slope U2 and the interception with the y-axis U are found by linear regression, see Fig. 6 (a, b). In the case of (a) and (b) we find: UL= 3.8 + 0.030 AT (red line).

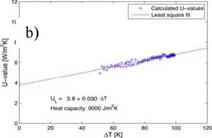The collector’s heat capacity is not accounted for in (a), hence the derived UL-values of the measurements in the morning and afternoon do not coincide. By choosing the heat capacity Ce=9 kJ/(m2 K) in (b) the AT-dependent heat loss coefficient from the morning and afternoon match. The chosen heat capacity Ce does not have a significant influence on the UL-values as long as the measurements before and after noon cover about the same AT interval. The U-values were determined for different tilt angles and the resulting collector efficiencies have been compared with earlier efficiency tests of the same collector. The presentation and discussion of these results would exceed the frame of the present work; it is referred to [19].

Fig. 6. U-value UL as a function of the temperature difference AT between the mean absorber temperature
and the ambient temperature if the collector’s heat capacity Ce is set to zero (a) and to 9 kJ/(m2 K). The line
represents the least square fit; results based on raw data of measurements performed at set-up A.

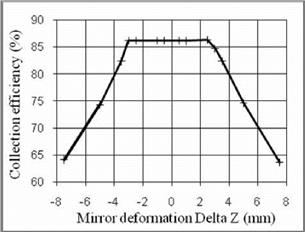After having introduced the procedure for mirror deformation reproduction, the first study examines the consequences produced by the deformations of a solar trough collector. The study is based on the use of ray tracing analyses that allow controlling all the optical parameters. Considering the application of sunlight exploitation, the most important features probably are collection efficiency, angular aperture and acceptance angle. The collection efficiency E is the ratio between the light focused on the absorber and the light received by the collector entrance aperture. The angular aperture of a collector represents the total aperture angle receiving the sunlight rays and it is usually expressed as Field Of View (FOV). While the acceptance angle is the limit aperture for which the collection efficiency keeps its maximum value. The angular analyses of the solar trough collector are discussed in Sections 4-5.

The collection efficiency of solar trough has been monitored in order to evidence how much it is affected by the geometrical deformation of mirror profile.

The configuration considered in this study is characterised by the following parameters: f=780mm, D=50mm,

G=70mm, T=2mm. The absorber centre is located in the focal point of the parabolic mirror.

The simulations are carried out for various values of the conic constant K,

corresponding to elliptic and hyperbolic Fig. 3. Deformation effects versus wrtiral

edge displacement.

deformations. The results are graphically reported in Fig. 3, whose calculation parameters are detailed in Table 1. The sampling for the conic constant K (Column 2) is not linear because we preferred to choose as reference parameter the deformation at the mirror extreme. The reference quantity chosen to represent the mirror deformation is the vertical displacement of mirror extreme Delta Z, reported in Column 6 of Table 1.

Column 1 indicates the deformation type (specifying the curvature direction of the deformation with reference to Fig. 2): elliptic (internal/up) or hyperbolic (external/down). Then Column 2 reports the value of each corresponding conic constant K.

The successive three columns of the table refer to the geometrical parameters of every deformed profile. Referring to Fig. 2, the point of mirror extreme has as coordinate (Ymax; Zmax), respectively reported in Column 3 and Column 5. Still referring to the semi-profile, half of the length of each deformed profile is in Column 4, confirming that the rigid deformation is calculated keeping almost unchanged the total length of the mirror. The calculation is approximated considering only integer values of mirror semi-aperture Ymax.

Finally the effect of mirror deformations is reported in Column 7 as collection efficiency values; the calculation has been carried out with linear sampling in Delta Z. Figure 3 visualises the variations of collection efficiency as a function of the mirror deformation parameter Delta Z.

The sign of the Delta Z values reported in Table 1 is in agreement with Fig. 2: the vertical edge displacement is positive in the elliptic case (int./up in Fig. 2) and is negative in the hyperbolic case (ext./down in Fig. 2).

In conclusion for the examined trough collector the effect of elliptic deformations is significant only for vertical edge displacement Delta Z > 2.5 mm.

While in the hyperbolic deformation case the effect of solar trough deformations is considerable only for absolute values of Delta Z > 3 mm.

Thermotropic hydrogels are chemically or physically cross-linked polymer networks which are poured with appropriate water content. At low temperatures the aqueous solution is dissolved homogeneously on the molecular level, so that a clear state is achieved. Above the switching temperature scattering domains are formed by an aggregation of the polymers and/or by separation of free water from the polymer network [2,3,7,8].

Numerous thermosensitive hydrogels exhibiting a cloud point are discussed in the literature. Examples are aqueous solutions of polyvinylethers [9], polyvinylalcohol [10], poly(N-substituted acrylamide) [11], poly(N-vinyl alkylamide) [12], ethyleneoxide-polypropyleneoxide [13], copolymers of N-vinyl — 2-pyrrolidone and hydroxyethylmethacrylate [14], poly(methyl-2-acetamidoacrylate) [15],

poly(methyl-2-acetamidoacrylate-co-methyl acrylate) [16], polyvinylacetone [17], poly(N-oxazolines) [18] or cellulose derivatives [4].

As to performance properties thermotropic hydrogels possess a high potential for solar collector applications. The materials are characterized by a high transparency in the clear state (>82%) along with low haze and a change in solar transmittance by 77% at temperatures adjustable between 5 and 100°C. The materials exhibit an excellent switching performance with a steep switching gradient, a high reversibility, and low hysteresis [4,19,20,21]. But as the transition is based on physical interaction between the components the materials have several problems with long-term stability and ageing. Furthermore the materials have to be UV protected [4]. The water makes the thermotropic hydrogel susceptible to freezing and limits the operation temperature range [21]. Thermotropic hydrogels filled into the intervening space of a double glazing, place high demands on sealing of the glazing. If not sealed properly the layer will dry out [2]. If synthetic materials are used thermotropic hydrogels are quite expensive. If biopolymers are used costs decrease significantly. However, the use of biopolymers requires a thorough protection against microorganisms [22].

A thermotropic hydrogel glazing, a sandwich of two glass panes and the encapsulated hydrogel, is announced by Affinity Ltd. (Japan) [2]. However, no thermotropic hydrogel is described currently that exhibits the working temperature range and switching temperatures required to provide adequate overheating protection for solar collectors. Further developments should focus on the adjustment of adequate switching temperatures (55-80°C) and the improvement of the long-term stability.

Advantages of a solar air collector system in general

• Air is for free.

• Air cannot freeze.

• No deterioration of the heat-transfer-fluid.

• No high pressure thrusts during a stagnation situation.

• No costs for secure systems, e. g. safety valves, membrane expansion vessels.

• No environmental or security problems in case of system leakages.

• Collector is intrinsically safe to itself and to the system.

• Solar air collectors enable easy and cost-effective highly scaleable solar thermal collector fields.

Disadvantages of a solar air collector in general

• Air has a lower heat capacity than water.

• Movement of air can cause aerodynamic noises.

• To achieve the same mass flow of air then of water, you need more electrical energy for the fan than for a water pump.

Our first prototype collector was installed in Freiburg, Germany in Dec 2005 [1]. The evaluation of measurements that were performed during summer 2006 confirmed the theoretically derived performance parameters [3].

In summer 2006 the second linear Fresnel process heat collector with 132 m2 aperture area (66 kWp, th) was installed on the roof of a building of the company Robur S. p.A. in Bergamo, Italy, to power a H2O/NH3 absorption chiller with 17 kWth nominal cooling power (5TR). Since September 2006 the solar cooling system is continuously operated and monitored.

[4] Present address: Institut fur Solarenergieforschung GmbH, Am Ohrberg 1, 31860 Emmerthal, Germany.

Equation (2) allows evaluating the total solar energy on the collector scope.

n

G = ^ Idt (2)

.=1

1.1.2 The actual useful energy

Equation (3) allows calculating the actual useful energy extracted to the solar energy.

Q = mCfAT = mcf ((out — T. n ) (3)

1.1.3 The instantaneous efficiency

Equation (4) allows calculating the instantaneous efficiency defined as the ratio of the actual useful energy extracted to the solar energy intercepted by the collector.

Q

AgG

1.1.4 Reduced temperature difference

When the temperature at the collector inlet is employed, the reduced temperature difference is calculated as:

1.1.5 Graphical presentation of instantaneous efficiency

Graphical presentation shall be made by statistical curve fitting, using the least squares method, to obtain an instantaneous efficiency curve of the form

П = П — a1T* — a2G(T*)

or

П = П — UT