At the given site, ambient temperatures fall below 2 °C at 20% of the year [4]. But even other regions for future applications of this technology may require freeze protection for the solar field. The intention to feed the solar steam directly into the existing distribution precludes the options to operate the solar field with refrigerants or antifreeze additives to the water. Another option is to decommission the plant during winter. Draining and refilling the system requires hardly any additional equipment installation. However, maintenance effort will be increased, and annual performance reduced. The latter could be avoided by an automatic draining and refilling system, at the expense of increased complexity and cost.

The proposed solution is circulation heating during times with danger of frost. First estimates indicate that the solar gain during winter will over-compensate the heating demand for freezing protection, subject to verification during the system operation and monitoring phase. Heat sources

for the freeze protection could be either steam or condensate, but also waste heat utilization or electric heating may be considered.

3. Conclusions and outlook

The layout and integration of direct solar steam generation for a process heat application with parabolic trough collectors has been planned for a demonstration plant, which is under construction and will start operation late 2008. This will be the first installation which allows test and evaluation of direct steam generation in an industrial environment. A monitoring program will be performed to validate the design assumptions and simulation models. The integration of a solar steam generator into the steam distribution of existing conventional installations can be a cost effective alternative to the retrofit of solar steam or hot water systems supplying individual low to medium temperature processes.

4. Acknowledgements

The authors gratefully acknowledge the financial support given to the P3 project by the Federal German Ministry for the Environment, Nature Conservation and Nuclear Safety (contracts No. 0329609A, 0329609B, and 0329609C).

References

[1] C. Vannoni, R. Battisti, S. Drigo (Eds.), (2008). Potential for Solar Heat in Industrial Processes, Task 33/IV booklet by IEA SHC and SolarPACES, published by CIEMAT, Madrid, Spain.

[2] C. Brunner, C. Slawitsch, K. Giannakopoulou, H. Schnitzer, (2008). Industrial Process Indicators and Heat Integration in Industries, Task 33/IV booklet by IEA SHC and SolarPACES, published by Joanneum Research, Graz, Austria.

[3] W. Weiss, M. Rommel (Eds), (2008). Process Heat Collectors — State of the Art within Task 33/IV, Task 33/IV booklet by IEA SHC and SolarPACES, published by AEE INTEC, Gleisdorf, Austria.

[4] T. Hirsch, K. Hennecke, D. Kruger, A. Lokurlu, M. Walder, (2008). The P3 Demonstration Plant: Direct Steam Generation for Process Heat Applications, Proceedings of 14th Biennial CSP SolarPACES Symposium, Las Vegas, Nevada, USA, March 4-7, 2008.

[5] K. Hennecke, J. Kotter, O. Michel, D. Peric, D. (2002): Solar Process Steam Generation for the Production of Porous Concrete. 11th SolarPACES International Symposium on Concentrated Solar Power and Chemical Energy Technologies, Zurich, Switzerland, September 4-6, 2002.

Javiera Valdivia, Jesfis Lopez-Villada, Joan Carles Bruno*, Alberto Coronas

Universitat Rovira i Virgili, CREVER — Group of Applied Thermal Engineering, Mechanical Engineering
Department, Avda. Paisos Catalans, 26, 43007-Tarragona, Spain

Corresponding Author, iuancarlos. bruno@urv. cat

Abstract

The use of power cycles driven by solar energy to provide the required mechanical energy to drive the high-pressure pump of Reverse Osmosis systems for desalination is an interesting alternative to the conventional electric systems. In this paper it is presented a model developed in Trnsys/Trnopt for the optimisation of the operating temperature in these systems to maximise the desalted water production. Two systems are studied and compared to provide the mechanical energy required for the Reverse Osmosis system: a steam Rankine and an organic Rankine cycle using n-pentane. The solar field consist of solar trough collectors. The complete solar field/organic Rankine cycle is modelled using Trnsys in the case of steam and an EES model linked with Trnsys in the case of the organic fluids. the results in terms of energy efficiency and production of desalted water are very similar operating the system at an optimal hourly temperature at the collector’s outlet or at an optimal daily temperature. However, significant differences where found between the optimal temperatures in winter and summer days. In the future the developed model will be extended to study also other types of solar collectors and working fluids.

Keywords: Reverse osmosis desalination, solar thermal energy, organic Rankine cycle

D. Ciobanu1*, I. Visa1* and D. Diaconesc1*

1 Transilvania University of Brasov, Centre for Sustainable Development, Eroilor Street, Brasov, Romania

* Corresponding Author, daniela. ciobanu@unitbv. ro

Abstract

Concentrator collectors are generally used in order to increase the amount of energy absorbed from the Sun. These collectors use only the direct radiation, being equipped usually with a single axis tracking system. The paper refers to parabolic trough collectors, whose geometry imposes one axis tracking system. In order to set the kinematic and dynamic condition necessary for the tracking system, it is considered as a math example the month of March when the average day time is of 12 hours. Thus, the reflector should rotate 1800 during the 12 hours. The maximization of system efficiency is obtained by operating the collector at specific moments aiming to minimize the energy consumption during the tracking generation.

The dynamic behavior of the tracking system is obtained based on the modeling and simulation of the mechanism by using ADAMS software.

Keywords: solar-thermal energy conversion, tracking system, cam mechanism, multibody system method

1. Introduction

The function of a solar collector is simple; it intercepts incoming insolation and turns it into thermal energy that can be applied to meet a specific demand.

Solar collectors are classified depending on the working principle in plate and concentrator. Plate collectors use the global radiation while the concentrator collectors use only direct radiation. Most common concentrator collectors are parabolic trough, Fig.1, a, central receiver and parabolic dish, Fig. 1, b, [1].

A parabolic trough concentrates the incoming solar radiation into a line running along the length of the trough, Fig. 2. A tube (receiver) carrying a transferring heat fluid, is placed along this line absorbing the concentrated solar radiation and heating the inside fluid [2, 3, 4].

For a better concentration of the solar radiation, due to the change of sun position on the sky, these collectors are equipped with tracking systems.

Tracking systems are classified by their motions. Rotation can be round a single axis (which could have any orientation but which is usually horizontal East-West, horizontal North-South, vertical, or parallel to the earth axis) or can be round two axes. The parabolic trough collectors are designed to operate with tracking rotation round one axis due to its geometry [1, 5].

Most common tracking systems use a gear box and a belt, [6, 7], rope or chain transmission. Collector trackers also use actuators or systems based on “hydro-mechanic”, [8] or gravitation, [9] principle.

Belt and rope transmission tracking systems require accurate maintenance and could generate errors; chain transmission cannot be used for large dimension collectors; systems based on “hydro­mechanic” principle have large dimension and those based on gravitation principle require a daily human intervention and are influenced by the environment temperature.

The proposed tracking system derives from a rotational (cardioids), [10] cam mechanism and oscillating role follower. A complex mechanism is generated by this, similar with a bolts gear. The mechanism uses two opposite cams and multi-followers generated by a wheel with two rows of bolts with half step delay. The advantages of this mechanism are: reduced dimensions by use of a two teeth gear — cams, lustiness, low costs due to a simple design, smooth motion and high efficiency due to the cycloidal gear, generating a more accurate operation comparing with other tracking systems. Equipped with a servo-engine and an adequate temporization, this mechanism can generate a controllable collector orientation.

First system tests determined the maximum achievable air temperature in the treatment chamber. Later testing verified that the solar system was able to match required heating/cooling ramps. Some typical T-t curves used in the ceramics industry were chosen as an example.

3. Results and discussion

The results of the most representative ‘maximum temperature test’ can be seen in Figure 4#, where the maximum achievable temperature with a solar irradiance of 980 W/m2 is 1000°C. It is worth mentioning that this limit is set by the receiver material, as its 1180°C safety limit was measured with the solar furnace shutter open only 85%.

The absorber material is a ‘SiSiC’ ceramic, chosen because of its proven availability and good performance in volumetric receivers at the PSA9.

When upper system limits had been determined, testing for facility suitability for complex T-t cycles was begun.

SolarPRO Test 060130
120
110
100
90
80
70
60
50
40
30
20
10
Fig. 4. Maximum temperature test

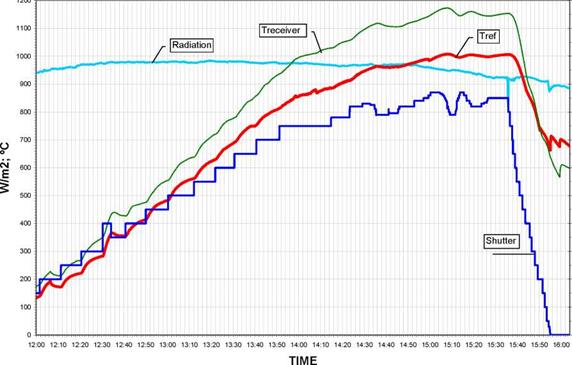
Temperature on receiver’s surface (°С)

Temperature in the center of the treating chamber (°С) Temperature of the theoretical T-t cycle (°C)

Direct solar radiation (W/m2)

Opening percentage of power attenuator (%)

The theoretical ceramic drying cycle T-t curve, in which a maximum temperature of 150°C must be achieved in 40 minutes, followed by a steep cooling ramp down to ambient temperature is shown in Figure 6.

As seen in Figure 5, there was no problem in following the required heating ramp. On the contrary, difficulties arose in accomplishing the desired cooling ramp. The air-cooling system may have to be specifically modified to increase the inlet cooling-air flow when necessary to solve this problem.

Fig. 5. Results of a drying test

Finally, ceramic baking was also tested in a higher temperature range, in which a maximum temperature of 800°C must be reached in 10 minutes, as seen in Figure 6. Test results were quite satisfactory.

Treceiver Temperature on receiver’s surface (°С)

Tref Temperature in the center of the treating chamber (°С)

Tcycle Temperature of the theoretical T-t cycle (°С)

Radiation Direct solar radiation (W/m2)

Shutter Opening percentage of power attenuator (%)

4. Conclusions

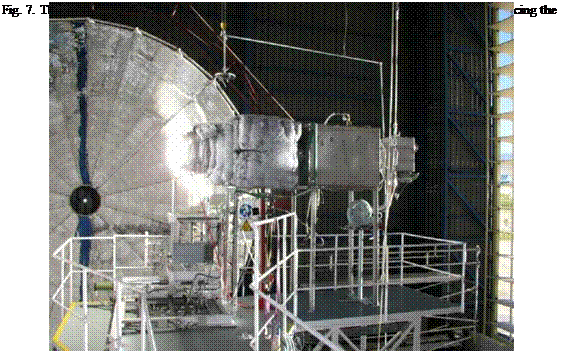
A project has been launched to study the feasibility of using concentrated solar energy for high — temperature industrial processes, such as ceramics manufacturing. A solar device (see Figure 7), based on the well-proven volumetric receiver technology has been designed, manufactured and assembled in the PSA Solar Furnace.

Preliminary testing has been performed to:

— Determine the maximum achievable air stream temperature.

— Study the feasibility of performing a ceramic drying cycle to industry requirements

— Study the feasibility of performing a ceramic baking cycle to industry requirements

Results so far are encouraging, as a maximum temperature over 1000°C has been reached in the air stream, and the system has been proven able to match the heating ramps quite well, which will allow all the selected processes to be studied.

Nevertheless, we are working in several aspects that still can be improved, and new results will be presented in future works.

The solar assisted heat pump desalination system, which has been constructed, is an experimental setup, thus it produces a small amount of desalinated water. In order to make it economically feasible, it is necessary to scale up the system into a higher production capacity, one that is able to provide water for domestic needs, or to supply water in remote areas. From Table 1, we can see that to supply the water requirement for a single family we need to produce at least more than 150 l of water per day. With a production rate of 150 //day, the system will also be able to supply

potable water to a remote elementary school of 60 students. However, we must always take into account the amount of investment needed and the payback period of the system to make it economically feasible.

A high price of solar liquid collector will cause the solar energy savings to be less competitive compared to conventional fuel systems. Therefore, it is necessary to make use of the heating and cooling provided by the heat pump efficiently. In order to achieve this, a new setup is proposed as shown in Figure 7. In the new setup, the refrigerant is allowed to condense fully through the distillation chamber and water tank, thus heating the feed water. Therefore, the need of liquid solar collector to preheat the feed water may be eliminated from the system. In the proposed new design, the distillation chamber will maintain the same vacuum pressure of 0.14 bar, thus water saturation temperature will still be at 52 °C. Refrigerant leaving the desalination chamber will preheat the feed water close to a temperature of 50 °C and feed water will be further heated by the electrical heater to 70 °C.

The proposed setup produces distillate water at different production rates, and thus different component sizes and investments are needed. A comparison of the production rates, 225 //day, 450 //day, and 900 //day showed that at lower production rate, the payback period increases. At low production rate, as shown in Figure 8, less solar energy is used in the process, thus the fuel savings is reduced, resulting in higher payback period. At 225 //day the payback period is close to 4 years, while at 450 and 900 //day the optimum payback period close to 3.5 years. At 900 //day, the operation and maintenance cost of the system is high, thus reducing the savings acquired from solar energy usage, causing a similar payback period to 450 //day. At 225 l/day the optimum collector area is 20 m2, while for 450 and 900 l/day it is 34 and 67 m2, respectively. For production of 450 // day, it is achieved with a compressor power input of 15 kW, and 30 kW for 900 // day.

As shown in Figure 9, installation of solar water collector to the system with a production rate of 900 //day will increase its optimum payback period to more than 4 years. A system with the same production rate will have a lower payback period without utilizing the solar water collector.

6. Conclusions

A series of experiments has been conducted with the solar assisted heat pump desalination system. A liquid solar collector was added to the system to preheat the feed water before entering the distillation chamber. Experimental results showed that the system could reach a Coefficient of Performance (COP) of 10, and at a relatively stable meteorological conditions, the evaporator collector has an efficiency value between 80 and 90%, whereas the liquid solar collector has an
efficiency value between 50 and 60%. The water production rate is generally close to 1 l/hr for the system, with a Performance Ratio (PR) close to 1.5.

An economic analysis was conducted to determine the feasibility of the system. It was found out that in order to be feasible, the system’s production capacity must be increased. However, the increase of production rate will also increase the investment cost of the system. With the high price of liquid solar collector, the payback period of the system becomes less feasible. A new design of the system, one that does not use liquid solar collector, was proposed. With the new design, the payback period of the system becomes much more attractive. A high production rate will require more investment cost, and it is shown that removing the liquid solar collector will reduce the payback period significantly. The optimum payback period of the system with a 900 l/day production is close to 3.5 years with a 67 m2 evaporator collector. Analysis of the economic parameters showed that the oil price is very influential in determining the competitiveness of the system.

In conclusion, it was found that the solar assisted heat pump desalination system exhibits great potential for future developments. With the increase of oil fuel prices, solar energy will likely to be more economically feasible as a source of clean energy.

Nomenclature:

I Solar irradiation, W/m2

AC Collector area, m2

Reference:

[1] Renato Lazzarin (2001), Ground as a possible heat pump source, Geothermische Energie, 32/33, 9, http://www. geothermie. de/gte/gte32-33/gte32-33index. htm

[2] T. N. Anderson, G. L. Morrison, M. Behnia (2002), Experimental analysis of a solar-boosted heat pump water heater with integral condenser, Proceedings of Solar 2002, Australian New Zealand Solar Energy Society

[3] J. Siqueiros & F. A. Holland (2000), Water desalination using heat pumps, Energy, 25, 717-729

[4] Onder Ozgener, Arif Hepbasli, A review on the energy and exergy analysis of solar assisted heat pump systems, Renewable & Sustainable Energy Review, 2005, pp.1-16

[5] Torres Reyes E, Cervantes de Gortari J., Optimal performance of an irreversible solar-assisted heat pump, Energy Internal Journal, 1(2), 2001, pp.107-111

[6] Hawlader, M. N.A., K. C. Ng, T. T. Chandratilleke, D. Sharma and Kelvin Koay H. L., 1987, Economic Evaluation of A Solar Water Heating System. Energy Conversion Management, vol. 27, pp 197 — 204

[7] Kreider, Jan F., Charles J. Hoogendoorn, and Frank Keith, 1989, Solar Design: Components, Systems, Economics, Hemisphere Publishing Corporation

[8] Hawlader, M. N.A., Prasanta K. Dey, Sufyan Diab and Chan Ying Chung, 2004, Solar Assisted Heat Pump Desalination. Desalination, vol. 168, pp 49 — 54

[9] Stein, Benjamin, John S. Reynolds, and William J. McGuinness, 1986, Mechanical and Electrical Equipment for Buildings 7th Edition, John Wiley and Sons

C. Alexandru*, M. Com^if and I. Vi§a

Transilvania University of Bra§ov, Product Design and Robotics Department, 29 Bd. Eroilor, 500036,

Bra§ov, Romania
* calex@unitbv. ro

Abstract

This paper presents researches on increasing the efficiency of the solar energy conversion, by designing and optimizing a single-axis tracking mechanism, which changes the daily position of the collector. The main task in optimizing the active component (the tracked solar collector) is to maximize the energetic gain by increasing the solar input and minimizing the energy consumption for tracking. The tracking system is approached in mechatronic concept, by integrating the electronic control system in the mechanical structure of the solar tracker at the virtual prototype level. Thus, the physical testing process is greatly simplified, and the risk of the control law being poorly matched to the real system is eliminated.

Keywords: solar panel, tracking mechanism, control system, virtual prototype

1. Introduction

There is a fact that the fossil fuels (gas, oil, coal) are limited and hand strong pollutants. In the last 15 years, the price of petroleum had tripled and the previsions on the medium term there are not quite encouraging. The increase of the emissions of carbon dioxide, responsible for the global warming and for the greenhouse effect, may have devastating consequences on the environment. The solution to the previously highlighted problems is the renewable energy, including the energy efficiency, the energy saving and systems based on clean renewable energy sources, like sun, wind and water. The solar energy conversion is one of the most addressed topics in the fields of renewable energy systems. The sun is a giant nuclear fusion reactor and the energy it supplies is equivalent of about 27,000 times the total amount of energy presently produced from all other sources. The present-day techniques allow converting the solar radiation in two basic forms of energy: thermal and electric energy. The technical solution for converting the solar energy in thermal energy is well-known: the solar collectors [1].

The efficiency of the thermal solar systems depends on the degree of use and conversion of the solar radiation. The energy balance refers to the surface that absorbs the incoming radiation and to the balance between energy inflow and energy outflow. The rate of useful energy leaving the absorber is given by the difference between the rate of incident radiation on absorber and the rate of energy loss from the absorber. In literature, the increasing of the efficiency of the solar collectors is approached mainly through the optimization of the conversion to the absorber level. On other hand, the degree of use of the solar radiation can be maximized by use of tracking systems for the orientation of the solar panels in accordance with the paths of the Sun. Basically the tracking systems are mechanical systems that integrate mechanics, electronics, and information

technology. These mechanisms are driven by rotary motors or linear actuators, which are controlled in order to ensure the optimal positioning of the collector relatively to the Sun position.

The orientation principle of the solar collectors is based on the input data referring to the position of the Sun on the sky dome. For the highest conversion efficiency, the sunrays have to fall normal on the receiver so the system must periodically modify its position in order to maintain this relation between the sunrays and the collector. The positions of the Sun on its path along the year represent input data for the design process of the tracking systems. The Earth describes along the year a rotational motion following an elliptical path around the sun. During one day, the Earth also spins around its own axis describing a complete rotation that generates the sunrises and the sunsets. The variation of the altitude of the sun on the celestial sphere during one year is determined by the precession motion, responsible for a declination of the Earth axis in consideration with the plane of the elliptic yearly path. In these conditions, for the design process of the tracking systems there are considered two rotational motions: the daily motion, and the yearly precession motion.

Consequently, there are two basic types of tracking systems: single-axis tracking systems, and dual-axis tracing systems. The single-axis tracking systems spins on their axis to track the sun, facing east in the morning and west in the afternoon. The tilt angle of this axis equals the latitude angle of the loco because this axis has to be always parallel with the polar axis; in consequence for this type of tracking system is necessary a seasonal tilt angle adjustment. The two-axis tracking systems combine two motions, so that they are able to follow very precisely the Sun path along the period of one year; that’s why dual axis tracking systems are more efficient than the single one, but also more expensive because they are using electrical and mechanical parts that determines the usage of expensive components in the system. Generally, for the orientation of the solar collectors, single-axis tracking systems are used.

Determining the real behaviour of the tracking systems is a priority in the design stage since the emergence of the computer graphic simulation. Important publications reveal a growing interest on analysis methods for Multi Body Systems — MBS [2, 3]. In the last decade, a new type of studies was defined through the utilization of the MBS software: Virtual Prototyping [4, 5]. This technique consists mainly in conceiving a detailed model and using it in a virtual experiment, in a similar way with the real case. One of the most important advantages of this kind of simulation is the possibility to perform virtual measurements in any point or area, and for any parameter. Thus, the behavioural performance predictions are obtained much earlier in the design cycle of the tracking systems, thereby allowing more effective and cost efficient design changes and reducing overall risk substantially.

In these conditions, our paper presents researches on increasing the efficiency of the solar collectors by designing and optimizing a single-axis tracking mechanism, which changes the daily position of the collector. The main task in optimizing the tracked solar collector is to maximize the energetic gain by increasing the solar input, and minimizing the energy consumption for tracking. The design is made by developing the virtual prototype of the mechatronic tracking system, which is a complex dynamical model. In fact, the virtual prototype is a control loop composed by the multi-body mechanical model connected with the dynamic model of the motor, and with the controller dynamical model.

For developing the virtual prototype, we used a digital prototyping platform, which includes CAD (CATIA), MBS (ADAMS/View), and C&C (ADAMS/Controls & EASY5) software solutions.

The approach is made in the concurrent engineering concept, by integrating the mechanical device model and the control system model at the virtual prototype level. In this way, the physical testing

process is greatly simplified, and the risk of the control law being poorly matched to the real (hardware) system is eliminated.

A solar concentrator with a fixed reflector has been developed. The first prototype of the collector consists in a 4.5 x 6 m reflector that concentrates the sun radiation on 8 rows of evacuated tubes (Fig. 12).

The experimental characterization of the collector is still under progress. By now the highest efficiency obtained for an inlet temperature of 100°C is 43% for a direct incident radiation of 890

W/m2. Although this thermal efficiency is low compared to the 70% of maximum optical efficiency obtained for normal incidence, it should be taken into account that it has been obtained for an incidence angle close to, but not coincident, with the normal, that the reflector was not cleaned, and that in the evaluation of the optical efficiency, structure shadows and edge effects were not taken into account. Therefore there is still room to optimize the design in order to approach to the theoretical limits, particularly in the areas of the upper structure design and the fine adjustment of the tracking. In the next months it is planed to further evaluate the collector under different radiation and weather conditions and to numerically simulate a detailed collector model using ray-tracing techniques. This will allow the identification of the primary sources of losses and will provide the fundamental guidelines to optimize the prototype.

5. Acknowledgements

This project has been funded by the CIDEM, an agency of the Generalitat de Catalunya (regional

government from Catalonia), and by a PROFIT credit of the Spanish Ministry of Industry, Tourism and

Commerce.

References

[1] European Commission, (2001) Green Paper — Towards a European strategy for the security of energy supply. Luxembourg: Office for Official Publications of the European Communities.

[2] POSHIP The Potential of Solar Heat for Industrial Processes Project No. NNE5-1999-0308. European Commission Directorate, General Energy and Transport.

[3] Russell, J. L, Jr. DePlomb E. DP. Gulf Oil Corporation. Concentrator, method, and system for utilizing radiant energy. US Pa. 3868823. 1975

[4] Pujol R. Martinez V. Moia A. Schwiger H. Analysis of stationary Fresnel like concentrator with tracking absorber. 13th International Symposium on concentrating solr power and chemical energy technology. 2006.

[5] Ravinder Kumar Bansal. Thoretical Analysis of fixed mirror solar concentrator. A thesis presented in partial fulfillment of the requeriments for the degree master of science in engineering. Arizona State University. May 1974

[6] Russell, J. L, Jr. Principles of the fixed mirror concentrator. General Atomic Company. San Diego, California. SPIE Vol. 85 Optics in Solar Energy Utilization. 1976.

[7] Russell, J. L. Jr.; Schuster, J. R.; Eggers, G. H. Development status of the fixed mirror solar concentrador. Proceedings of the 12th intersociety energy conversion engineering conference. 1141-1146. 1977.

[8] Pujol R. Analisis de un concentrador solar lineal con reflector fijo y foco movil siguiendo una trayectoria circular. A final paper presented for the degree of Master of Physics. Universitat de les Illes Balears. September 1974.

[9] Martinez V. Pujol R., Moia A. Schwiger H. Analysis of a stationary parabolic linear concentrator with tracking absorber. 13th International Symposium on concentrating solar power and chemical energy technology. 2006.

The reactor chamber is a cylindrical cavity made of S30815 (ASTM) / 1.4835 (EN) heat-resistant steal that contains a windowed aperture. Manufacture of the solar-receiver started at the beginning of this year following the design of ETH [1]. The cavity-receiver has been divided into four segments for assembly reasons. Fig. 1 shows the pre-assembled solar-receiver at PSA installations.

An insulation liner is included to protect the steel housing from the very high temperature inside the reactor, this insulation is able to withstand up to 1400 °С. The liner is fabricated with a studied combination of two types of ceramic materials based on Al2O3 (89 to 95 % Al2O3). Additional insulation will be applied at the outside of the reactor.

The feedstock (slurry) is injected into the reactor’s cavity via a tangential inlet port located near the window. The window is actively cooled and kept clear from particles and/or combustibles gases by means of an aerodynamic protection curtain created by a tangential flow of steam through four tangential nozzles located at the conical part of the aperture.

The most important features regarding the receiver reactor are related to the injection of the coke slurry (without using a inert gas) and the reactor window.

One of the most important issues of this study has been to design an efficient injection system with regard to their availability to fluidise reactants into the cavity without the need of a carrier gas. The mode of conveyance of the material and the gasifying medium, in traditional gasification, is presented by general categories of: fixed-bed, fluidised-bed and entrained-bed reactors. In particular, in the entrained flow gasifier, coal and other solid fuel particles concurrently react with steam and air in suspension fluid flow mode. The full entrainment of the particles requires relatively high gas velocities and these lead to high throughputs and residence times of only a few seconds.

For this cavity reactor, an entrained flow has been simulated. An innovative concept using a combined slurry / pneumatic transport system was used. The pulverized coke is mixed with the (liquid) reactant water that is subsequently evaporated to create a high-speed “pneumatic” injection. The feasibility of this concept was stu

demonstrated in the 5 kW solar reactor at PSI [3]. This system allows to fluidize particles up to 100 microns without the need of a carrier gas. Inside the cavity, the mixture forms a flow that progresses towards the rear path and exit the cavity via an axial outlet port located at the back of the cavity.

On the second hand, up scaling of the reactor quartz window with a diameter of 1400 mm is of particular challenge. To find out the real limits of this window, a comprehensive finite elements calculation has been performed.

A. Bridgeman* and S. J. Harrison

Solar Calorimetry Laboratory, Queen’s University, Department of Mechanical and Materials Engineering,

130 Stuart Street, Kingston, ON, K7L 3N6, Canada

Corresponding Author, Bridgeman@me. queensu. ca

Abstract

An indirect solar assisted heat pump (ISAHP) system for heating domestic hot water has shown promise as an alternative to conventional electric or natural gas water heaters. In a previously conducted theoretical study, it was concluded that an ISAHP could operate with a lower life-cycle cost than a conventional solar domestic hot water (SDHW) system. Therefore, to further investigate the feasibility of the proposed system, an experimental study was conducted on a prototype (ISAHP) system. To undertake the study, a fully instrumented heat pump water heater was assembled in a laboratory environment and connected to a simulated “solar heat” input. The “solar” input was provided by an electrically heated circulation loop that delivered temperature-controlled fluid to the heat pump evaporator. This allowed repeatable test sequences to be performed in the laboratory regardless of weather conditions. A simulated solar profile ranging from 750 — 1500 W was delivered to the heater throughout the test. The corresponding fluid temperature ranged from 15 — 37°C, and the results indicated coefficient of performance (COP) values ranging from 2.4 to 3.2. These results, while in close agreement, are approximately 12% lower than those predicted from previous theoretical values.

Keywords: Solar assisted heat pumps, Heat pumps, Domestic water heating, Canada

1. Introduction

In Canada, water heating is the second most energy intensive end use in the residential sector, accounting for 22% of the consumed energy [1]. Due to growing concern for depleting fuel supplies, higher fuel prices and greenhouse gas emissions, alternatives to the conventional water heating methods such as electric and natural gas water heaters are being investigated. Two systems currently receiving considerable attention worldwide are Solar Domestic Water Heaters (SDWHs) and heat pump systems that source energy from the ambient air, or geothermal energy.

While each of these systems may operate with lower energy consumption than a typical electric water heater, both systems have performance limitations. Air-source heat pump water heaters are attractive in temperate regions, but lack popularity in Canada due to the warm temperatures needed for their proper function [2]. Geothermal heat pumps demonstrate improved performance over air source heat pumps because the heat is drawn from the earth, which is much warmer than ambient in the winter. However, due to the ground loops necessary for these types of heat pumps to function, property alterations and high initial costs have made them less practical for existing homes [2]. Solar Domestic Water Heaters have been increasing in popularity in Canada, and can decrease the energy consumption of an electric water heater by up to 90% in the summer [3], but large temperature differences between the collector and ambient air during the winter months lower the collector efficiency significantly, limiting the seasonal performance.

A combined system, known as a Solar Assisted Heat Pump (SAHP) could be used to alleviate many of the disadvantages of either system operating independently. The advantage to the heat pump cycle, by coupling it with a solar thermal collector, is an increase in evaporator temperature over either air-source or ground-source heat pumps. This increase in temperature results in an improved heat pump coefficient of performance (COP). From the solar collector point of view, the use of the heat pump lowers the fluid temperature returning to the collector near or below ambient. This lower temperature increases the collector efficiency, and allows for substantial heat gains with low cost unglazed solar absorber panels, even under marginal conditions [4, 5, 6]. The combined system allows for efficient operation over a wider range of seasons and weather conditions, and for more hours throughout the day.

The concept of a SAHP dates back to 1955 when it was first proposed by Sporn and Ambrose [7]. Numerous studies took place in the 1980s and early 90s examining the feasibility of SAHP systems for either space or water heating. Most of these systems were classified as Direct Expansion Solar Assisted Heat Pumps (DX-SAHP), in which the refrigerant would flow through the solar collector directly, which doubled as the evaporator for the heat pump. Chaturvedi [5, 8] found that collector efficiencies between 40 — 70% were feasible with bare collectors operating under ambient condition in winter, and found heat pump COPs ranging from 2 — 3, which was 30 — 50% higher than air source heat pumps. In the mid 90s Morrison [6] stated that the majority of previous systems proposed had not achieved commercial success due to the complexity of the combination of heat pump and solar collector components, and high installation costs due to the need for plumbing, electrical and refrigeration connections during installation. He then proposed an integral design, in which the collector and heat pump unit were incorporated as an integral part of the storage tank, which must be installed outside. Morrison found only a slight decrease in performance compared to a typical system in Sydney, Australia, but predicted a substantial reduction in cost, and simplification of installation. Huang and Chyng [9, 10] have recently investigated similar integral DX-SAHP systems in Taiwan. They found COPs reaching up to 3.83 during a long term performance test, in which the system was run for 13,000 hours continuously.

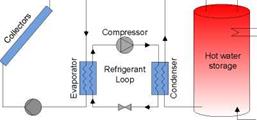
Although the integral DX-SAHP overcame installation complexities of SAHP systems and achieved commercial success in some parts of the world, installing the water storage tank outdoors introduces another problem in the Canadian environment. The cold conditions in the winter months increase the heat loss from the tank decreasing the system’s performance. To avoid this problem, an Indirect Solar Assisted Heat Pump (ISAHP) is under investigation at the Queen’s Solar Laboratory in Ontario, Canada. A schematic of an ISAHP is shown in Figure 1. This system differs from a direct solar assisted heat pump in that the heat pump collects energy via a heat exchanger connected to the collector anti-freeze loop, rather than flowing through the collector itself. This eliminates the need for long refrigeration lines and costly refrigeration fittings on the collector, but allows for the heat pump unit and storage tank to be installed inside the residence. Another feature of this system is the external side-arm natural convection heat exchanger, which acts as the heat pump’s condenser. As the heat exchanger transfers energy from the refrigerant to the potable water, the water increases in temperature causing its density to decrease. This induces buoyancy driven natural convection, circulating the water through the heat exchanger and eliminating the need for a pump. Due to the relatively low flow of the natural convection loop, this configuration has the potential for increasing thermal stratification in the storage tank. The benefit of stratification is that it delivers cool fluid from the bottom of the tank to the heat pump condenser, while maintaining hot water at the top of the storage for distribution to the load. This improves the overall system performance [11].

1st Internatio /

To Load

The application of renewable energies such as solar energy to produce fresh water is receiving increased interest due to the need for solving the water shortage problems in various areas of the world at the same time as conventional energy sources used for obtaining water in different scenarios become depleted.

The world desalination installed capacity has increased from a bit more than 10 million cubic meter per day in 1986 to more than 42 million in 2006. Only in Spain the capacity of newly commissioned plants in 2006 was higher than 400 000 m3/day [1]. So it is not surprising that the use of renewable energy sources in water desalination is of high interest, especially for remote areas where a conventional energy supply is not easily available. This application is, however, still not well developed and it has only been tested in pilot plants and at a few demonstration sites. Numerous examples of research work on renewable energy desalination systems can be found in [2-5] as well as in other reviews in the literature. There are various desalination methods available on the market that use mainly thermal or mechanical energy in their fundamental separation processes. Among them,

Reverse Osmosis (RO) is quite suitable for small to medium capacity systems and also has good perspectives for cost reduction and improvement in efficiency in the near future [6]. In RO, pressure applied to the saline solution forces pure water through a semipermeable membrane. The membrane is selective and allows the passage of water but is impermeable to other substances.

The energy to produce the required pressure for RO can be generated with renewable energy sources such as wind energy, dish-Stirling systems, solar thermoelectrical plants or photovoltaic solar electrical generation. Solar thermal energy coupled to a power cycle by using direct mechanical power can also be employed.

Water is commonly used in Rankine power cycles, although other types of inorganic (ammonia, ammonia/water, …) and organic fluids (hydrocarbons, fluorocarbons, siloxanes, …) can be used. The main advantage of organic working fluids in Rankine cycles (ORC) is that they can be driven at lower temperatures than similar cycles using water and also in many cases superheating is not necessary.

The research on ORC has been focused in the production of electricity, mainly related to recovery of low temperature waste heat, geothermal heat, biomass, or solar energy. Many references to these applications are available in the literature. A couple of facilities with ORC plants using solar thermal energy were constructed in 1978 in Cadarache, France and in 1981 at El Hamrawin, Egypt, but unfortunately there has been little information published about them [7]. A commercial parabolic trough ORC power plant (Saguaro plant) completed in 2006 in Arizona is of particular interest. It is a 1 MWe plant using n-pentane as the working fluid for the ORC, and is based on plants used in geothermal applications having 10340 m2 of parabolic trough collectors [8]. Studies on ORC applications for desalination are very scarce although a few projects exist and some studies are available. Burgess and Lovegrove [9] discussed the application of solar thermal powered desalination using membrane and distillation technologies. One of their conclusions was that more detailed analyses of solar driven RO are required to determine its costs and applicability. In [10] it is proposed a solar ORC system using R-134a and evacuated tube collectors. The system efficiency is low (7%) but the authors considered it comparable to equivalent photovoltaic desalination systems. The first laboratory test simulating the heat provided by solar collectors has been given by Manolakos et al [11]. An screening and performance assessment of working fluids for RO solar thermal desalination can be found in Delgado [12]. In Bruno et al [13] it is presented a model optimising the solar field/ORC global efficiency using a process simulator and calculating the required solar field area for several types of collectors and selecting the most appropriate fluid for each one. Also a technical and economical comparison with a photovoltaic/RO system is presented. The main conclusion was that solar driven systems specially those using medium-high temperature collectors can compete favourably in terms of specific annual cost €/m3 with the PV/RO system.

Using medium-high temperature solar collectors e. g. trough collectors, the use of water or organic fluids is possible, e. g. the Solar-Thermal power plant Andasol (Spain) using water or the Saguaro solar plant (USA) using n-pentane. In summary, existing research on solar ORC for desalination is very limited, and few efforts have been reported on determining the most useful working fluids for this application and their optimal operation conditions.

In this paper it is presented a rigorous model developed in Trnsys/Trnopt [14] linked with EES [15] for the optimisation of the operating temperature of solar Rankine cycles connected to RO desalination

plants to maximise the desalted water production. Two cases are presented and compared to provide the mechanical energy required for the RO system: a steam Rankine cycle and an organic Rankine cycle using n-pentane. The selected thermal solar field consist of trough solar collectors because in previous preliminary studies made by the authors [13] it was concluded that they provided the higher global efficiency. The complete solar field/organic Rankine cycle is modelled using Trnsys in the case of steam and Trnsys for the solar field and an EES model for the ORC system linked with Trnsys in the case of the organic fluid. The performance of the RO system is calculated using the ROSA software [16] and the modelling parameters used in [13].