4.1 Results

Experiments have been conducted on the desiccant wheel with the parameters (e. g. outside temperature, outside humidity ratio, regeneration temperature and regeneration humidity ratio) varying in the range defined in the section 2. The complete DOE of 4 parameters operating between 2 levels needs 24=16 experiments. When a combination of the studied parameters was

difficult to achieve experimentally (3 experiments) the results of the model was used to complete the DOE. Table 1 below shows the results

Table 1. Dehumidification rate of the desiccant wheel for different operating conditions Ti w1 T8 W8 w1-w2 T1 w1 T8 w8 w1-w2 [°C] [g/kg] [°C] [g/kg] [g/kg] [°C] [g/kg] [°C] [g/kg] [g/kg] 25 11 55 10 4.8 35 11 55 10 2.9 25 11 55 15 3.6 35 11 55 15 1.7* 25 11 75 10 6.8 35 11 75 10 5.2 25 11 75 15 5.8 35 11 75 15 4.1 25 14.5 55 10 5.4* 35 14.5 55 10 4.1 25 14.5 55 15 4.3 35 14.5 55 15 2.95 25 14.5 75 10 8 35 14.5 75 10 6.7 25 14.5 75 15 7 35 14.5 75 15 5.6* * Calculated by the model

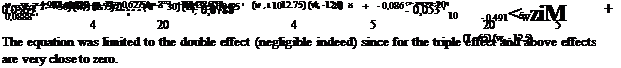
Solving 16 equations for the effects identified in the equation (1). The dehumidification of the desiccant wheel is then written in function of the operating parameters

4.2 Analysis

Form the equation identified we notice a constant dehumidifying effect of 4,987 g/kg which will be increased or decreased depending on the operating conditions. In order to compare the effect of each parameter with the constant effect, they are divided by this later and a graphical comparison can thus be established. The figure below shows the effect of each parameter.

As commonly known, figure 3 shows tha the regeneration temperature has the most significant impact on the dehumidification performance of the desiccant wheel. In the same time outside conditions has an important impact on too.

• Increasing the regeneration temperature from the mean value (65°C) to its upper limit will increase the dehumidification 23%

• Increasing the outside temperature to its higher limit will decrease the dehumidification of 17%.

• Increasing the outside humidity ratio will increase the performance of the desiccant wheel of 13%

• Increasing the regeneration humidity ratio will decrease the performance of the desiccant wheel of 8%.

The reason behind these observations is the vapor pressure difference of the air and that of the surface of the silica gel. This vapor pressure difference is the driving force of the adsorption phenomena. Reminding that if the desiccant is cold the vapor pressure at its surface. For the moist air the temperature does not have a significant impact on the vapor pressure while humidity ratio does.

The desiccant coming for the regeneration sector is hot and dry, it is first cooled down by the outside air in the process sector and then the adsorption occurs.

Fig. 3 Effect of the operating conditions and their combinations

So if we increase the temperature of the outside air its vapor pressure will not increase significantly in reversal it will not cool sufficiently the hot desiccant form arriving from the regeneration sector yielding a high vapor pressure at the surface of the desiccant and thus the adsorption will be less efficient. If we increase the humidity ratio of the outside air its vapor pressure will increase significantly yielding a higher vapor pressure difference thus increasing the adsorption. When increasing the regeneration temperature, the regeneration air vapor pressure does not increase significantly while the desiccant is heated and its vapor pressure increases leading to an efficient drying of the desiccant. This desiccant leaving the regeneration sector is dry and thus having fewer vapor particles, yielding a low vapor pressure at its surface and thus high adsorption. When increasing the regeneration humidity ratio we will increase the vapor pressure of regeneration air stream, reducing the transfer from the desiccant to the regeneration air, yielding less drying of the desiccant. When the sorbent will leave the regeneration with more water vapor at its surface its vapor pressure is then higher, reducing thus the adsorption.

This clearly shows the limitations of the desiccant cooling technique regarding outside conditions. It demonstrates that high outside temperature reduces significantly the performance of the desiccant wheel. Regarding the outside humidity ratio even if the dehumidification increase with increasing outside humidity ratio, we noticed that for outside temperature beyond 30°C the maximum dehumidification rate is 6 g/kg. Taking into account the maximum humidity inside the building (e. g. 11.8 g/kg) and the humidification across the supply humidifier we conclude that the maximum outside humidity under which a desiccant system will operate efficiently is 14.5 g/kg.

3. Conclusion

In this paper a sensitivity analysis based on the design of experiments was conducted on a desiccant wheel using mainly experimental results. A numerical model was validated experimentally and provided the missing combination of the experiments. The impact of the outside and regeneration conditions on the dehumidification rate of the desiccant wheel was studied. As widely known the regeneration temperature has the most significant impact on the dehumidification rate, but the impact of the outside temperature, outside humidity ratio and the

regeneration humidity ratio are very important as well. These results showed that desiccant cooling is an interesting option for moderately hot and moderately humid climates

Nomenclature

Cpa	heat capacity of air [J. Kg-1.K-1]	NUT	number of transfer unit [-]
cpv	heat capacity of water vapour	RMSE	Root mean squared error
	[J. Kg’.K-1]		[unity of the variable]
cpm	heat capacity of the regenerator matrix	t	time [s]
	[J. Kg-‘.K-1]	T	temperature [K]
C	heat capacity (J. Kg"1.K"1)	T a	air temperature [K]
Fi	potential characteristic [-]	T eq	equilibrium temperature [K]
ha	enthalpy of moist air [J. Kg-1]	Tm	matrix temperature [K]
H	enthalpy of the desiccant [J. Kg-1]	u	fluid velocity (m. s-1)
Jt	lumped heat transfer coefficient [s-1]	Wa	humidity ratio of moist air. [Kg/Kg]
Jm	lumped mass transfer coefficient [s-1]	Wd	water content of desiccant [Kg/Kg]
Le	Lewis number [-]	z	coordinate in the flow direction [m]
ma	air mass flow rate [Kg. s-1]	T	ratio of matrix mass over air mass [-
Md	mass of the desiccant matrix [Kg]	n	efficiency [-]
Mm	mass of the aluminium matrix [Kg]	p	density [Kg. m-3]
N	angular speed of the wheel [rd. s-1]	Yi	parameter [-]

Acknowledgments

The authors would like to thank Mr Michel Burlot for his valuable technical support on the experimental

installation. This work was supported by ADEME (French Agency of the Environment and Energy

Management) and the regional council of Poitou Charentes.

References

[1] P. Bourdoukan., E. Wurtz., P. Joubert., M. Sperandio,. (2008). Potential of solar heat pipe vacuum collectors in the desiccant cooling process: modelling and experimental results. Solar Energy. 0.1016/j. solener.2008.06.003

[2] J. Goupy, (2001). Introduction aux plans d’experiences, Dunod.

[3] P. J. Banks, (1972). Coupled equilibrium heat and single adsorbate transfer in field flow through a porous medium — 1. Caracteristics potentials and specific capacity ratios. Chemical Engineering Science, 27, 1143-1155.

[4] I. L. Maclaine-cross, P. J. Banks, (1972). Coupled heat and mass transfer in regenerators — prediction using analogy with heat transfer. Int. J. Heat Mass Transfer, 15, 1225-1242.

[5] J. J. Jurinak. (1982). Open cycle solid dessicant cooling — component models and system simulation, Ph. D, Winconsin, Madison.

[6] P. Stabat, (2003). Modelisation de composants de systemes de climatisation mettant en oeuvre l’adsorption et l’evaporation d’eau, Ph. D, Ecole des Mines, Paris.

[7] W. M. Kays, A. L London, (1984). Compact Heat Exchangers, McGraw-Hill, New York.

[8] SPARK. (2003). Simulation Problem Analysis and Research Kernel. LBNL, Berkeley, California.

[9] P. Bourdoukan., E. Wurtz., P. Joubert., M. Sperandio, (2008). Critical efficiencies of components in desiccant cooling cycles and a comparison between the conventional mode and the recirculation mode."

In proceedings of ECOS conference, Krakow, Poland, 1, 435-443