The FracTherm® program is to be improved especially with a focus on practical aspects concerning both production and operation of the collector. This concerns the algorithm itself as well as the implementation of additional features. The further program development can increase the number of possible geometries and thus the flexibility in designing absorbers. An example for an improvement is the possibility to use different bifurcation geometries, which can have an important mechanical and hydraulic impact on the resulting roll-bond absorbers.

2.1. Adaptation of the roll-bond process for the production of solar absorbers Nowadays roll-bonding is basically used — and optimized — for the production of evaporators for refrigerators. However, the requirements for a solar absorber are different. Therefore it is necessary to find out the restrictions of roll-bonding such as the maximum width and height of channels as well as the minimum radius of bifurcations depending on the internal pressure. It has to be checked whether common dimensions of roll-bond channels can be changed in order to gain more flexibility in design and reduce the pressure drop. The effect of different bifurcation geometries has an important impact on mechanical and hydraulic behaviour of the absorber. The minimum radius of roll-bond structures is one important limitation, because it has an influence on the minimum distance between channels. Currently the use of circular constructions is common in roll-bond panels. Mattheck has shown that notch stresses of circular notches can be reduced dramatically by shape optimization based on load — adaptive growth which was observed in nature (trees, bones). In [5] Mattheck shows that a significant reduction of notch stresses can be obtained even with the pure graphic “method of tensile triangles”. It is very likely that the mechanical stability — and thus the flexibility in design — can be increased if roll-bond geometries are changed from circular to optimized shapes. Different bifurcation geometries are shown in Fig. 3; a), b) and c) are already implemented in the FracTherm® program, d) is not yet (the shape in the figure is generated manually). The different geometries are to be investigated experimentally within the project.

The easiest method is to use wood screws which can pierce the aluminium and screw into the wooden foundation board. These can be manually applied or an automatic screwing machine can be used.

7. Example of MEGASOL installation.

A traditional Scottish cottage with a slated roof was chosen to install a MEGASOL solar water heating collector. Firstly the slates were removed from the area devoted to the collector to leave the bare wooden boards covered with thin roofing felt. Then a layer of reflective bubble polythene sheet was fixed over the roofing felt. Then a grid of thermally enhanced EPDM pipes (1mm wall thickness, 15mm outside diameter) was fixed to the insulation, with spacing between pipes of 150mm. The pipes were formed into U loops with both flow and return manifolds at the same end. The pipes were temporarily fixed in place with adhesive tape. Then standard size aluminium sheets of 1mm thickness were laid on top of the pipes and screwed through the aluminium to the wooden substrate with wood screws. Then the aluminium sheets were painted with matt-black paint (non-selective). Finally, sheets of twin — wall polycarbonate were located over the absorber plate, with a 25mm air gap, and fixed by standard glazing bars. Air gaps at the top and bottom of the sheets were sealed up. The EPDM pipes were connected to 22mm diameter flow and return manifolds by 15mm stubs. These pipes were then connected into the building’s central heating system. Pictures of the collector in construction and finished are shown in figs 2 and 3 below.

The whole collector was constructed by a two workmen who had no previous solar experience. The collector area was 25m2 and the total cost of materials and construction was approx EU2,500, giving a cost per unit area of approx EU100/m2. This is believed to be unusually low.

8. Collector efficiency

Thermal analysis of the above described collector has been undertaken and gives Fr tau. alpha= 0.64 and Fr U= 4W/m2K.

9. Pressure resistance

Burst tests indicate that the collector should be able to handle pressures of up to 4bar.

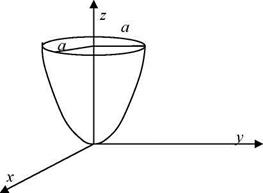
We can choose different shapes for the static concentrators useful surfaces. Using some software which allow the simulation of the light rays way is convenient. For this paper we chose a paraboloidal concentrator.

The rotation paraboloid equation is (Fig.1)

x2 + y2 — 2a2z = 0 (1)

where, a is the radius of the circle obtained by intersecting the paraboloid with the xOy plane at the distance z=1.

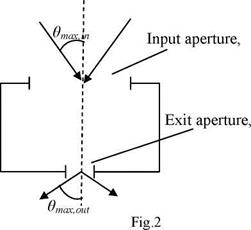
In the Fig.2 is being shown the generalized scheme of a concentrator: Ain = Aconc is the area of the light input aperture, Aout = Arec is the area of the exit aperture, 6max, in is the maximum incidence angle, on the entrance aperture, at which it produces the concentration, 6max out is the maximum angle on which the radiation falls on the receptor (PV cell).

The maximum concentration optic factor is [2,8,9]

where, n is the refraction index of the environment in which the cell is immersed (for air n=1). For incidence angles between 0 and 6max, in, the optic concentration factor is equal with the ratio between the radiant flow density on the receiver, Brec, and the radiant flow density on the input aperture, Bconc

b

C = — (3)

optic

conc

The geometric concentration factor is

A

C = — (4)

geom

A out

The concentrator’s performances are determined by the optical efficiency, Ioptic, which is defined as a proportion between the optic concentration factor and the geometric concentration factor

optic

C

The Sun’s declination, 5, at the solar noon in a day of the year, n (n = 1,365), is calculated with the equation

S лі 23,45 • 284 + п

S[rad ] = п———— sin(2n————— ). (6)

180 365

In the equation (6), n is calculated with the equation [10,11,12] n = 30.416(1 -1) + x, (7)

where: 1 is the month (1 = 1,12) and x is the number of the day of the month.

The incidence angle, в, of the radiation on the surface inclined with the 5 angle, orientated with the azimutal angle у is [13,14,15]

cos# = sin8sinфcos5 — sin8cosфsin5cosy + cosScosФcos5cos® +

(8)

+ cos 8 sin ф sin 5 cos Y cos ® + cos 8 sin 5 sin Y sin ® •

where Ф is the latitude of the place.

The hour angle ® is given by the equation

where TS is the solar time (solar hour) and T0 is the solar noon, t0 = 12.

The solar hour is defined as

TS = TL + E — 4Ol — Vl ). (10)

In the (10) equation, (pL is the legal longitude, (pl is the place longitude, TL is the legal hour and E is the time equation,

E = 2.292(0.0075 + 0.1868cos /3 — 3.2077sin /3 — 1.4615cos2^- 4.089sin2^), (11)

where the angle в is

P[rad ] = ^ (n -1). (12)

365

For s=0, we obtain the zenithal distance 0Z,

cos#z = sin8sinф + cos8cosфcosa> (13)

At ground, the solar intensity, B, is

B = S(1 + 0.0034cosn)a• exp(- d/Z0^Q ) (14)

where: S =1353 W/m2, a = 0.88, d = 0.28.

On a surface, A, with random orientation, в, the radiant flow density is

BA = B cos# (15)

If the radiation concentrated from the A] on the A2, the concentrated flow density is

Ba2 = Ba1 • A1/ A2 (16)

The quantity of diurnal solar energy incident on the input aperture of the concentrator has been calculated using the equation:

Qconc =YjnR 2 BconCi АТ i=1

where At is the incrementation step of time, n is the number of temporal sequences with the width At in one day. The quantity of solar energy diurnal incident on the output of the concentrator (photovoltaic cell) has been calculated using the equation:

Qrec =Y. W 2 Breci At (18)

i =1

The efficency of the concentrator has been calculated using the equation:

n = Qrec / Qconc (19)

K. Resch1*, R. Hausner2 and G. M. Wallner3

1 Polymer Competence Center Leoben GmbH, RoseggerstraBe 12, A-8700 Leoben, Austria
2 AEE Institute for Sustainable Technologies, A-8200 Gleisdorf, Austria
3 Institute of Materials Science and Testing of Plastics, University of Leoben, A-8700 Leoben, Austria

Corresponding Author, resch@pccl. at

Abstract

The main objective of the present study was to evaluate the potential of thermotropic layers to provide overheating protection for solar collectors by theoretical modeling of an all-polymeric solar panel. The investigations showed that for a collector with twin-wall sheet glazing and black absorber the stagnation temperatures can be limited by the use of thermotropic glazing. For maximizing the collectors efficient working temperature range the thermotropic layer should exhibit switching temperatures between 50 and 60°C. The impact of the thermotropic layer on collector efficiency is low, as long as the solar transmittance in the clear state is above 0.85. A residual solar transmittance ranging from 0.25 to 0.60 in opaque state is effectual to control the stagnation temperatures in the range from 80 to 130°C.

Keywords: all-polymeric flat plate collector, overheating protection, thermotropic layers

1. Introduction

Polymeric materials offer a significant cost-reduction potential for solar thermal collectors and may thus benefit a broader utilization of solar energy for various heating purposes. However, conventional solar thermal collectors reach stagnation temperatures up to 200°C, which exceed the maximum operating temperatures of cost-efficient plastics (~80-130°C). As especially polymeric absorbers made of cost-efficient plastics tend towards irreversible deformation and/or degradation when exposed to elevated temperatures for prolonged periods an appropriate overheating protection is required. The energy flux in all polymeric flat-plate collectors may be controlled by using thermotropic layers [1,2]. Thermotropic glazings change their light transmission behavior upon reaching a certain threshold temperature reversibly by switching from a transparent to a light diffusing state [3].

Within the present study theoretical modeling is applied to evaluate the potential of thermotropic layers to provide overheating protection in an all polymeric flat-plate collector. Specific focus is given to the effect of the thermotropic materials on overall solar collector performance as well as to required material properties, such as solar transmittance in clear and opaque state, switching temperature and switching performance to limit the stagnation temperature to 80-90°C, which is the required maximum operating temperature for domestic hot water and space heating applications. Furthermore the frequency distribution of stagnation temperatures for a collector with thermotropic overheating protection is determined.

2. Modeling

C. Nunes1 , M. J. Brites1, J. F.Mendes1, M. Lopes Prates1, J. Alexandre1, M. C. Ferreira1,
A. J.Martins2, V. Teixeira2, J. Carneiro2, M. J. Carvalho1

1INETI — Estrada do Pago do Lumiar, 1649-038 Lisboa, Portugal 2Universidade do Minho — Centro de F^sica, Campus de Gualtar, 4710-057 BRAGA, Portugal * Corresponding Author, clarisse. nunes@ineti. pt

Abstract

This paper presents results of application of natural and synthetic organic pigments in selective solar absorber coatings. Some of these photo excite pigments were tested like solar radiation absorber pigments in paint coatings and others as a form to increase the absorption of solar radiation in titanium oxide monolayer selective coatings produced by reactive magnetron sputtering. Morphologic aspects and optical properties are discussed as a function of deposition parameters and of formulation parameters. The main goal is to prepare solar absorber surfaces as a tandem of TiOx thin films, organic pigment using a conductive substrate or of paint with organic and/or inorganic pigments and conductive substrate, all reaching great photo thermal conversion efficiency, high durability and low costs to the ending product and stability at medium range temperatures (120-150°C). The best optical properties for titanium oxide sputtered films were 88% for solar absorption, with 7% of emissivity for deposition parameters of: pulsed frequency 200kHz, reverse time of 0.4ps, discharge current of 0.7A, argon flow rate of 50ml/min and oxygen flow rate changing from 0 to 2.5ml/min. The results obtained with paints were not satisfactory. The best couple values for solar absorption and emissivity were respectively 94%, and 74%.

1. Introduction

In this paper we present some results of organic pigment application in selective solar absorber coatings developed within a Portuguese research project [1], obtained as a tandem of TiOx thin films, organic pigment and conductive substrate, described elsewhere [2, 3]. This idea is a spin off of the work done by members of the research team in the field of TiOx thin films deposition by reactive magnetron sputtering in DC or pulsing DC mode over conductive substrate [4], and of synthesis and characterization of [60] and [70] fullerene-coumarin dyads [5, 6], in a parallel way as that done to develop dye-sensitized nanocrystalline TiO2 thin films for PV applications [7], and to develop paints with inorganic or organic pigments [8, 9, 10].

Although many and good work has been done in the area of selective solar absorber surfaces, still subsists interest to develop selective solar absorber surfaces, with high durability, low costs and thermal and environmental stability, to apply in solar thermal conversion, mainly for higher values of medium range temperatures (120-150°C). Our idea is that titanium oxide films could be a good stake, because of high stability, no toxicity and possibility of wide range of refraction index variation [11-12].

It’s known that optical properties of materials are dependent of factors like the composition (electrons distribution in atomic external energy levels, minimum energy necessary to change for places of higher energy, type of link between atoms and molecules, minimum energy to cause inter or intra-molecular vibrations), the structure (that determine the strength of link between atoms and molecules and consequently the movement of more external electrons), the morphology (interfaces

between columns or grains could be scattering places of radiation, and shape, size and orientation relatively to incident radiation are also determinants in scattering effects and radiation interference phenomena’s, the same happens with defects, displacements, discontinuities, hols and them shape and size), the surface topography (adequate roughness could be motive of multiple reflections of incident radiation, with some absorption at each incidence and also forcing radiation to come into material) and the presence of different layers with their interfaces (which could cause interference phenomena’s motivated by adequate conjugation of thickness with composition layer and level of package, determinant in values of refraction indices, being optical thickness equal to physical thickness multiplied by refraction index, etc [13]).

Having in attention described topics, titanium oxide films obtained by magnetron sputtering seems to be adequate option for selective solar absorption coatings once that refraction index can be changed with adequate control of deposition parameters, between certain limits, to control composition, package, thickness, number of different layers, structure, morphology and topography of coatings [13-17].

2. Experimental details

A carton paper overhanging part is added to the bottom of the selective coated collector. An artificial suction effect is tried to be constituted by using two fans. In this case, air pressure in the chambers is increased but isn’t enough to provide air flow by convection. As seen in Table 1, the surface temperatures of the collector are variable. The surface temperature values are rather high in the middle part of the collector. So increase of the temperature and velocity of the incoming air from bottom of the collector are unproportional. Consequently air exit from the top of the collector is unrealized by reason of air flow losses.

Marco Bakker1, Jochem Nijs2, Wim van Helden1, Angele Reinders2
1 ECN, Energy Research Centre of the Netherlands, P. O. Box 1, 1755 ZG Petten, The Netherlands,
telephone: +31 224 56 8079, fax: +31 224 56 8966, email: m. bakker@ecn. nl
2 University of Twente, Faculty of CTW, Department of Design, Production and Management,
P. O.Box 21, 7500 AE Enschede, The Netherlands

Introduction

In 2030, the energy demand of newly built houses will be fully covered with solar energy, according to the vision of the European Solar Thermal Technology platform. Naturally, this should not interfere with the increasing demand for indoor comfort and the need for affordable accommodation. This places a lot of demands on future solar thermal collectors.

With the materials and production technologies that are currently being used, it is not possible to make the transition to a next generation of solar collectors with a strongly improved price/performance ratio. New collector concepts are required, based on new materials, new production technologies, and on increased intelligence of the collector system. In this paper we will illustrate how new concepts for solar collectors have been developed with a focus on implementation in 2030.

Backcasting

Within WAELS, a Dutch national long-term research project, a vision on the solar thermal collector of the future was developed. To start as unbiased as possible, a backcasting method was used. This method starts with a future vision, and describes the developments between then and now that are needed to reach that future.

A series of three workshops was organised to get an overview of the developments in the various sectors related to solar thermal collectors. Experts from a. o. construction, installation, materials science, and information technology were invited to discuss the developments in their fields of expertise. These developments were extrapolated and combined in order to define a set of opportunities (e. g. new materials, sensors, construction technologies, etc.) and boundary conditions (e. g. evolved energy demand, competing technologies, etc.) for solar thermal collectors in 2030.

The main trends that were found as a result of the workshops—aspects that will become increasingly important for solar collectors—were:

•f lexibility: both building and installation can easily adapt to newly added components or to changes in user demands;

• integration: building and installation components are fully integrated and interwoven;

• intelligence: the building can independently adapt its behaviour to the ambient conditions and the user’s demands and behaviour;

• modularity: building and installation components are easily exchangeable;

• independence: the building can provide in its own energy needs.

High market penetrations have pool absorbers of (a) rigid, extruded sheets of polypropylene (PP) with intrinsic channels, Fig. 6 (a). Common are also flexible absorber mats of ethylene propylene diene monomer (EPDM) rubber pipes, here in red in order to match the colour of roof tiles (b).

Further examples of pool absorbers with pipe structure are plain polyethylene (PE) pipes (d) and ripped PP pipes (e). Fig. 6 (c) and (d) illustrate two examples of rigid, blow-moulded absorbers in high-density polyethylene (HD-PE): (c) flat panels of 200 x 110 x 1.5 cm and (f) pool absorber cassettes with honeycomb structure of 30 x 30 x 3 cm. Both absorbers can be interconnected in a matrix, parallel and in series. Commonly, pool absorbers are placed on the top of flat or slightly tilted roofs, various sophisticated solutions for fixing and preventing the collectors from displace­ment by wind, rain and snow exist.

2.1 Glazing

The glazing has to sustain the temperature gradient between the collector inside and the ambient temperature, solar irradiation, load of weather impacts due to wind, snow, hail and rain. Compre­hensive work on the durability of polymeric glazing has been performed, e. g. [8, 9, 10]. UV-resistant, thermotropic and anti-soiling coatings for polymeric surfaces are e. g. studied in Subtask C of IEA-SHC Task 39.

The aesthetics of the collector glazing is essential from an architectural point of view. Especially when large parts of a building’s facade or roof are covered by solar collectors, the integrated de­sign, size, shape, surface structure, colour and reflectance will contribute to the building’ s appear­ance. Rather common and known from the building and construction sector are twin-wall sheets of polycarbonate (PC) as collector cover (Fig. 7 (a)). Fig. 7 (b) shows a solar air collector with a PC twin-wall sheet as cover. Different collector cover designs are dome structures of PC (c), corru­gated roof coverings with 6 mm twin-wall structure of PC (d), and acrylic glazing of an integrated storage collector (e). The polymeric glazing in the examples (c) and (d) cover conventional, selec­tively coated metal absorbers with pressurised solar loop and water-glycol mixture as heat carrier.

The present study has three objectives, i) to investigate the temperature reduction in polymeric collectors by passive ventilation, ii) to demonstrate a built-in, temperature triggered mechanism to initiate the ventilation process iii) to present a simplified method for determining the temperature dependent heat loss coefficient and the heat capacity of glazed polymeric solar collectors from stagnation measurements. All measurements are performed under stagnation conditions (no heat carrier in the absorber). Central issues for the investigations in the present work are:

• Non-selective absorbers of polymeric materials (low temperature performance plastics);

• Building integrated installations;

• Solar heating system design: non-pressurised, water as heat carrier, drain-back function;

• Simple control system design, self-protective overheating control;

2.1. Polymeric collector

For the design of a polymeric collector it is important to have a high system performance, which is competitive with conventional collector systems. Due to the overall system design, the present system is not operative for absorber temperatures typically above ~90 °С, the heat carrier drains back to a drain-back reservoir and the absorber is filled with air. The present absorber material is a modified PPE/PS blend [12] and studied e. g. in [13, 14].

Here, the maximum temperature reduction due to ventilation is studied, however not whether the reduced stagnation temperature is suitable for a long service life of the polymeric absorber. Still it can be indicated that the stagnation temperature in the present collector should be significantly below 130 °С; the system design should be such that this temperature is only reached during short periods of time.

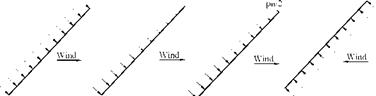
The tracker is equipped with a PV panel with a surface 1.48×0.67 m2, approx. 1 m2. The maximum wind speed for the Bra§ov region is 30 m/s resulting a maximum wind force on the panel Wmax = 580 N for a maximum wind pressure pm = 580 N/m2 [3]. The wind direction can be considered towards the front of the panel (fig. 2, a.. .c) or towards the back of the panel (fig. 2, d.. .f). Different assumptions can be considered on the distribution of the wind pressure on the panels: uniform pressure [4], resulting bigger wind force (fig. 2, a, d); trapezoidal distribution, approximating the distribution presented in [5], reversed for opposite wind direction (fig. 2, b, e); triangular distribution [6], reversed for opposite wind direction (fig. 2, c, f). For all six wind load cases presented in fig. 2, the load can be reduced to a single wind force W and a moment M, placed on the axis of the panel, with values according to Table 1.

pm pm

Fig. 2. Wind load (a — wind case 1; b — wind case 2; c — wind case 3; d — wind case 4; e — wind case 5; f —

wind case 6).

The only weight that is considered for preliminary design is G = 250N, the weight of the panel together with all the parts (frame) directly attached to it.

Table 2 presents the loading cases given by the extreme positions of the tracking system.