The system employed a small capacity fan, 12 DC 5We PAPST that required an external energy supply. The power needed was supplied by a small PV-panel unit of 10 We. Thus the performance of the fan was not continuous and therefore the flow rate was mainly depended on the irradiance levels. Thus for the highest irradiance level, the collector delivered the maximum flow rate and for lower irradiance levels, there was a limit where the fan did not operate.

The study of the solar collector performance requires the analysis of the flow rate dependence with the irradiance but also the pneumatic system characteristics. The fan was powered by the PV-panel that pumped the flow through the system to cross the woodchip. In order to find the relationship between flow rate and irradiance, it was necessary to study the pneumatic characteristics of both fan and system, and also the electrical connection between fan and PV-panel separately.

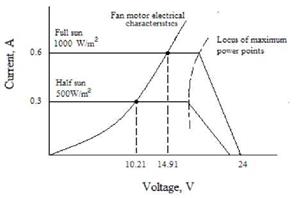The electrical connection between PV-panel and fan can be analysed in the I-V curves that describes the electrical characteristics of both 10We PV-panel and 5We fan, Figure 5 shows operational voltages at two irradiances levels. It may be noted that not all the power generated by the PV-panel is supply to the fan as operational points don’t coincide with the maximum power curve. During the periods of high irradiance, the fan will be operated over capacity, although the fan motor showed no signs of fatigue or damage. [6]

Fig 5: PV module and fan electrical characteristics with systems operating voltage

The final drying flow depended on the pneumatic characteristics curves of the system. The flow blown by the fan needs to overcome the pressure drop associated to the air resistance to pass through the solar dryer. The head losses of the solar dryer were located in three differenced parts: the transpired plate, the flexible duct and the woodchip layer. Therefore the drying flow depended on the type of plate used and on the thickness of the woodchip layer. For all the tests, the thickness

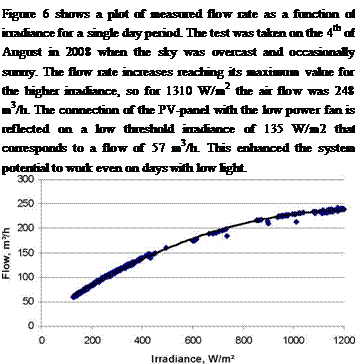of the woodchip layer was 3cm that corresponds to the 3kg of product employed before in the drying tests.

Fig 6: Measured flow rate vs. irradiance

Figure 7 shows the collector outlet and collector temperature rise against the irradiance for the same day as previous. Considering that air flow rate increases with irradiance, the graph shows the tendency of temperatures to increase with irradiance as well. For low irradiances the average temperature rise was 10°C and for high irradiance levels it was 20°C. The degree of scatter in the temperature rise is mainly attributed to variances in the ambient temperature and fluctuations in the collector flow. Also the transitory time to the steady state and wind effect may affect in the collector air and absorber plate temperatures [11].

The concept of the “two loop “system is different from the compact system and will be favourably from the economical point of view for daily capacities higher than 1000 litre. A sketch of the principle set up is given in figure 5. The design keeps four main differences compared to the compact systems:

A thermal storage tank is used to enable an extended operation time of the MD-modules even after sun-set ^Decreasing specific module costs

The system consists of two loops. The desalination loop is operated with sea water and is separated from the collector loop which do not have to be sea water resistant ^ Cheaper standard components (storage, collectors, pumps) can be used

Several MD-modules are operated in parallel.

A controller is used for charge and discharge of the heat storage and controlling a set point temperature for the evaporator inlet ^The operation conditions can be optimized regarding performance conditions of the MD process.

Simulation computations were carried out for the system design and the development of an adapted control strategy for two different pilot plants. The design capacity for the Aqaba system was 700 — 900 l/day and for the Gran Canaria system 1000 — 1600 l/day. Table 1 provides the key data for both systems.

Figure 6 shows a picture of the collector field (left hand side) and the heat storage, hydraulic board and the desalination unit (right hand side) of the Aqaba system installed in December 2005. The two loop system in Gran Canaria was installed in March 2006.

2.

Experimental investigations Compact System

The graphs in figure 7 (left side) represent one day of operation of the Compact System in Freiburg on 16th July 2006. The solar irradiation (Irrad) in the collector plain increases up to 1000 W/m2 at noon. The feed pump switches on when a set point temperature of 55°C at the collector outlet is reached. The feed mass flow is in the beginning about 300 l/h and increase with rising solar irradiation to a maximum of 500kg/h at noon.

The distillate production starts immediately after the system start up and increases continuously with the rising evaporator inlet temperature and feed volume flow. The maximum distillate mass flow during noon is about 25 l/h. The maximum evaporator inlet temperature is almost 90°C. The temperature at the condenser inlet (Tcond_in) increases during the day, due to the brine recirculation to the feed tank. At 12:00PM the refilling of the feed storage starts initialized by reaching the critical feed temperature of 50°C at the condenser inlet. As can be seen the temperatures (Tcond_in) decrease immediately by 10 K. The total daily distillate production at the 16th July 2006 was about 140 liter.

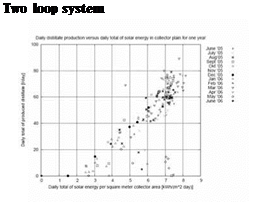
The first Compact System was installed in Gran Canaria in December 2004 and is still in daily operation with the first MD-module. Figure 6 (right hand side) presents a part of these long term measurements in Gran Canaria. The daily distillate gain is plotted versus the cumulated daily gain of solar energy. The considered period starts in the middle of June 2005 and ended in the middle of June 2006. As can be seen from the one year measurements there is no decrease of specific energy demand during the observed period. For example a daily solar gain of 7kWh/m2 enables an average distillate production of 60 l/day in June 05 as well as in June 06. Differences, in both directions, occur due to specific weather conditions an operation conditions.

Fig 7: Daily measurement — Compact System Freiburg (left), Long term measurement Gran Canaria — daily
permeate production vs. sum of daily solar irradiation (right)

A plot the operation performance of the two loop system in Gran Canaria is presented in figure 8 for a fine day in March. The “Irrad” graph represents the global radiation on the tilted collector surface. The “TCol_out” graph is the collector outlet temperature and the “T_evap_in” line represents the evaporator inlet temperature as adapted by the control unit. As can be seen the collector outlet temperature and the evaporator inlet are rising comparably until 11:00 AM (IG=500W/m2) when the set value at the evaporator inlet is reached. Then the collector outlet temperature continues rising while the evaporator inlet is set to 80°C.

From 6:30 PM to 7:30PM the controller switches to storage discharge. As can be seen the temperatures are fluctuating and can not be controlled to the set value. The reason can be found in the slow reaction time of the control unit respectively the slow movement of the Valve. From 7:30PM to 22:30AM the temperature control operates successfully again and the evaporator inlet temperature is set again to 80°C until the storage top temperature decreases below that value. The system is operated with a decreasing evaporator inlet temperature and decreasing distillate flow until 4:15AM, March 24. Then the switch of temperature of 58°C at the evaporator inlet is reached. The distillate volume flow during operation on the set point temperature is about 75 and 80 kg/h. The cumulated distillate gain from the operation period between 10:00 AM March 23 and 6:15 AM March 24 is 1240kg.

The specific energy consumption of the MD unit is in the range of 260 kWh/m3 distillate for low evaporator inlet temperatures of 55°C and decreases down to 180kWh/m3 distillate at a set point operation temperature of 80°C. The specific energy supplied by the collector — respectively the storage loop is 14% to 23 % higher than consumed by the MD unit. That difference can be considered as system losses.

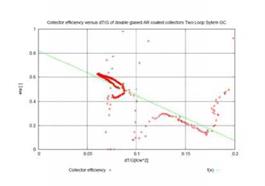
The diagram on the left hand site of figure 8 presents the collector field efficiency (nth) of the Gran

Canaria two-loop system. It was calculated from the temperature difference between collector field in — and outlet, the collector loop mass flow rate, the specific heat capacity of water (Cp) and the measured global radiation in the collector plain.

nth = (T_coll_out — T_coll_in)*mp_coll*Cp / IGt

It can be seen from the graph that in the range of standard operation between 0.06<(dT/G)<0.08 (for G =1000W/m2 and Tamb=20°C this is equal to an average collector temperature range of 80 — 100°C) the collector field efficiency is between 0.61 and 0.5. The stagnation temperature for G=1000W/m2 and Tamb=20°C can be calculated from the efficiency curve with 200°C.

In many cases it is not only more economic but also more efficient to stop the solar system under high irradiance than to store every single Joule which is not used soon and would get lost in the tank later. Therefore our principal concept is to avoid large storage tanks. This is even more the case where high temperatures are required that are accompanied by higher heat losses of the tank. On the other hand systems with small or without tanks can deliver solar heat much faster under bad irradiation conditions if the heat capacity of a storage tank is not a barrier.

The thermal standstill is a normal thing for water systems. In future the restarting of water systems from the thermal standstill will also be a matter-of-course as soon as heat is needed again. A storage capacity of 0.5 kWh per m2 collector area is transferred on the roof and can be saved with the storage tank. This is about 1/100 of the annual energy harvest or even more. A solar collector system that can be restarted from standstill at any time promises a much higher annual energy harvest than installations which can only leave stagnation by cooling down through natural heat losses.

With these positions Paradigma differs essentially from the official recommendations which are based on the traditional flat plate collector technology.

If the thermal process heat demand in industrialised countries is analysed, one recognises that there are two ranges of temperatures with a high industrial heat demand: one at a temperature range of 800°C and one in the range up to 250°C. This latter temperature range offers good conditions for the application of solar thermal collectors to cover at least part of that heat demand. The following industrial sectors are especially concerned:

• food and beverage industry

• breweries

• laundries and tanneries

• textile industry

• chemical industry

• paper and pulp industry

• investment goods industry

All over Europe, the process heat demand in this temperature range is estimated to about 400 TWh per year. The potential for solar process heat is estimated in [2] to be 90-112 GWth for the EU25 countries. The development of this market potential will open new growth segments for the European solar thermal industry. Moreover, very promising possibilities for the export of new solar thermal technology exist world-wide, especially to countries and regions with high solar irradiation. Therefore it is necessary to develop new appropriate collectors, system components, storage tanks and complete solar process heat systems in the next years. The aim should be to achieve a number of ‘good practice examples’ within the next years, so that in the future an increasing part of the process heat demand can be covered by solar thermal technology.

All results presented in this paper were simulated using solar thermal collectors integrated into the south facade (collector tilt angle = 90°). Heat losses from the building can be reduced because the effective u-value of the wall with integrated collector is reduced even when solar radiation is low. In addition, overheating of the collectors during times of stagnation in summer can be avoided.

Pedro Horta1*, Joao Farinha Mendes1, Agustfn M. Delgado-Torres2

1 INETI — Instituto Nacional de Engenharia Tecnologia e Inovagao, IP, Departamento de Energias Renovaveis, Estrada do Pago do Lumiar, 22, 1649-038 Lisboa, PORTUGAL Tel.: +351 217 127 099, Fax.: +351 217 127 195

2 Departamento Fisica Fundamental y Experimental, Electronica y Sistemas, Facultad de Fisica, Universidad de La Laguna, Avda. Astrofisico Fco. Sanchez s/n, 38206 La Laguna, Tenerife, SPAIN Tel.: +34 92 231 8102, Fax.: +34 92 231 8228 * Corresponding Author, pedro. horta@ineti. pt

Abstract

Project POWERSOL [1] aims at the “development of an environmentally friendly improved- cost shaft power generation technology, based on solar thermal energy, optimized for supplying basic need to rural communities”, bringing solar thermal mechanical power/electricity generation to a smaller scale (power outputs up to 500 kW) and allowing the use of cheaper and simpler optical systems, suitable for applications with lower requirements of maintenance and operation expertise, as those found in industry, desalination or small communities.

In the present article, a performance analysis of such a power system is presented, allowing a preliminary assessment of its design. The paper addresses the use of different solar collector technologies in the production of heat, in a range of top cycle temperatures between 150°C and 250°C, suitable for both stationary and single-axis tracking solar collectors.

The use of heat storage and backup systems, allowing extended cycle operation, is also assessed. System performance is evaluated after yearly operation simulation for different locations, enabling an evaluation of the most suitable design for different locations and operation conditions.

Keywords: solar thermal energy; small scale power generation; solar organic Rankine cycle

1. Introduction

The production of electricity or mechanical energy by means of solar energy conversion, has gathered, in the most recent years, a research effort proportional to the increasing interest of such technologies, as a solution covering the needs of either small and isolated communities or large scale power plants.

Whereas the photovoltaic conversion of solar radiation is a well established technology for electricity production, the technologies based in the thermal conversion present a growing number of applications, especially large scale plants based in different types of high concentration systems.

Nowadays, solar thermal driven mechanical energy production is mainly focused in large scale steam solar power plants. Yet, at the present, the use of the steam cycle in low power units or for top cycle temperatures under 350°C is not feasible, for either operational or economical reasons. In

such cases, the Organic Rankine Cycle (ORC) can be an alternative, given its relative high thermal efficiency and the use of dry fluids, impeding vapour condensation at turbine outlet [1-6].

Within the framework of the EU funded project POWERSOL [1,6], a solar thermal driven ORC is being considered, aiming the development of a small scale mechanical power generation technology. The mechanical energy produced could be converted into electricity and/or be used in desalination, water pumping or cooling. The heat rejected in the cycle could also be used — improving the exergy efficiency of the whole system — for water and space heating or cooling.

The core of the power system is an Organic Rankine Cycle (ORC), whose application in power generation at low temperature is well known, with a number of waste heat, geothermal and biomass based examples. In relation to solar thermal — driven ORC’s, there is a lower number of experiences, being the most representatives the Coolidge Solar Irrigation project [2] and the Small Communities Project [3] both developed at the beginning of the 80’s and the recently 1-MW solar thermal power plant operated by the Arizona Public Service in Saguaro (E. E.U. U.) [4]. Angelino et al.[5] also give a number of low power output solar heated ORC systems.

Three different top temperature levels are considered in the POWERSOL project, corresponding to different solar thermal collector technologies: cycle 1 — 80°C (flat plate collectors, FPC); cycle 2 — 150°C (compound parabolic collectors, CPC); and cycle 3 -200°C-250°C (parabolic trough collector and linear Fresnel concentrators). Solar collector prototypes are being developed specifically for each temperature level and a small ORC pilot plant will be erected in the PSA [7].

Considering a preliminary dimensioning of the overall system components (solar field, heat storage, ORC), results for yearly performance of both cycles 2 and 3 are presented in this paper, for three different locations: Almeria (Spain), Cairo (Egypt), Moura (Portugal), considering a system of 100 kW gross power output with different heat storage capacities, operating with or without a conventional energy backup.

C. M. Rangel1*, R. A. Silva1, T. I. Paiva1 and V. R. Fernandes1

1 INETI, Department of Materials, Campus do Lumiar do INETI, 1649-038 Lisbon, Portugal
* Corresponding Author, :armen. rangel@.ineti. pt

Abstract

Nanostructured semi-conductor materials based on titanium dioxide, with effective photo-catalytic properties under UV illumination, were synthesized and characterized with the objective of studying the photo-catalytic hydrogen production from water. The need to decrease the electron — hole recombination rate was accounted for by metal doping. Ethanol was used as a hole trap. Aqueous suspensions of the semiconductor powders, with noble metal loadings (Pt) were used for a selected catalyst concentration. Hydrogen production was found to be linear with UV irradiation time at near room temperature. pH variations during hydrogen production were followed and associated to the formation of acetic acid during the reaction.

Keywords: hydrogen production, doped-titanium dioxide, ethanol, photo-catalytic materials.

1. Introduction

The generation of hydrogen from water splitting using photo-catalytic surfaces of oxide materials has been recognized since the early seventies [1]. In the last decades interest in semiconductor photocatalysis has grown significantly, with works mostly referring to uses in water/air purification.

The photo-catalytic production of hydrogen by means of irradiation of a suspension of semiconductor oxides, presents attractive features over other methods with higher cost such as water electrolysis.

Some of the materials properties and requirements for solar hydrogen production include tailored electronic structure: band gap — essential for absorption of solar energy; and flat band potential — must be higher than the redox potential of the couple H+/ H2. Furthermore, efficient charge transport is necessary since low electrical resistance is required as well as effective charge separation and prevention of electron-hole pair recombination.

Titania is the base catalyst material of choice, notwithstanding the stability and non-corrosive properties, and the environment friendliness and low cost, the actual efficiency in the production of solar hydrogen is still very low, due to electron-hole pair recombination [2-6] and also due to TiO2 band gap (~3.2 eV) which only allows utilization of UV light.

The feasibility of photo electrochemical generation of solar hydrogen requires that the energy
conversion efficiency goes from current levels < 1% to levels of > 10%, with accompanying

durability. In order to increase efficiency in the use of semiconductor electrodes in electrochemical photolysis, integrated systems including semi-conductor / redox couples interfaces, deposition of metallic co-catalyst, sensitizers, etc. have been studied [2,3]. The modification of TiO2 properties may contribute for a more efficient hydrogen production that may take advantage of visible light utilization [4,7,8,9]. Effective charge transfer from water molecules and the TiO2 lattice requires the presence of surface active sites, associated to point defects, that can form activated complexes with water molecules.

In this work, a nanostructured semi-conductor material based on titanium dioxide, with effective photo-catalytic properties under UV illumination, was used for hydrogen production using ethanol as a sacrificial agent, with excellent results.

2. Experimental

A photochemical reactor with a total volume of 4.40 litters distributed between an internal (irradiated) reactor and an external reactor (fluid reservoir) was used according to need; a sensing pH electrode was allowed for as well as facilities for titration of H+, in order to adjust pH, when required. The internal reactor was contained in a black box and used a 450 W Hg immersion lamp (A. C.E. Glass Incorporated, NJ), as a radiation source. The emission spectrum of the lamp indicated that the UV radiation is mainly situated between 313 and 366 nm. Circulation between reactors, when required, was ensured by a peristaltic pump, according to the required recirculation rate. Agitation by magnetic stirrers in both reactors was also used.

In this paper, titanium dioxide Degussa P-25 was modified by photochemical deposition of Pt. Platinised TiO2 catalyst (at 1.5 wt.% Pt) was prepared using hexachloroplatinic acid (Riedel-de Haen) as the precursor. A pre-determined amount of TiO2 was first suspended in hot water and the hexachloroplatinic acid previously dissolved in an aliquot of fresh distilled water was added, with continuous nitrogen purging (15 min.) inside the described photo-reactor. The mixture was irradiated for 60 min, at constant temperature (30-40°C), to ensure that all the platinum in the suspension was reduced and deposited onto the surface of TiO2. The TiO2 / Pt catalyst was subsequently recovered by filtration and washed repeatedly with water. Finally, the powder was dried at 70°C and stored under vacuum in a desiccator. Catalyst were heat treated at 440 °C during one hour.

After some preliminary studies a concentration of TiO2 of 0.5 gL-1 was selected as well as a concentration of ethanol of 5 M and an initial pH of 11[10].

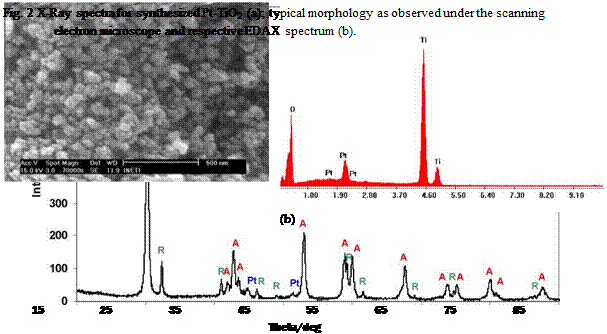
Characterisation of the powders was done by X-Ray diffraction using a Rigaku, model D-Max IIIC and by scanning electron microscopy (SEM) using a Phillips model XL 30 FEG microscope coupled to EDS.

3. Results and Discussion

In this work, a nanostructured semi-conductor material with effective photo-catalytic properties under UV illumination was synthesized using a photochemical method, taking as a base Degussa P-25 powder and platinum salts.

X-Ray diffraction data of the synthesized powder indicated the presence of anatase and rutile, see figure 2a). Anatase content was estimated to be ~ 89.21% and the crystallite size 21 nm. Typical morphology of the powder is shown in figure 2b), exhibiting particle size at a nanoscale.

Aqueous suspensions of the semiconductor powders, with noble metal loadings (Pt) of 1.5 wt% were used and the hydrogen production studied, for a fixed amount of the catalyst of 0.5 g/L. A 5M concentration of ethanol was used.

Typical results obtained during the photocatalytic production of hydrogen by UV illumination of the suspension of TiO2-Pt are shown in figure 3. The rate is presented in terms of the number of mol per unit time of produced gases by gram of catalyst.

A titration confirmed the presence of large amounts of acetic acid, indicating that pH variations during the reaction must be significant. Typical pH variations with irradiation time were measured starting from the initial pH value of 11 falling to 5.1, see figure 3.

Where Me — Metal (Pt); h — hole

Methane and CO2 can be produced by the decomposition of acetic acid, h + CHaCOO" ^ CO2 + CH30 (6)

Another pathway for CH4 formation may be the hydrogenation of CO2 according to equation (7)

CO2 + 4 H2 ^ CH4 + H2O

Another possible reaction pathway is the involvement of adsorbed surface hydroxyls on TiO2 in the trapping of holes. The interaction of surface hydroxyl groups with holes will result in the formation of hydroxyl radicals which, in turn, will interact with C2H5OH or its intermediates adsorbed on the metal — TiO2 surface or present in the vicinity to produce CO2 and other side products.

Another possible reaction pathway is the involvement of adsorbed surface hydroxyls on TiO2 in the trapping of holes. The interaction of surface hydroxyl groups with holes will result in the formation of hydroxyl radicals which, in turn, will interact with C2H5OH or its intermediates adsorbed on the metal — TiO2 surface or present in the vicinity to produce CO2 and other side products.

Work proceeds with analysis of the gas mixtures by gas chromatography.

Work in progress includes comparison with own synthesized sol-gel titanium dioxide modified with Pt in the similar experimental conditions. Modification of the titanium dioxide band gap is also in progress, in order to account for the advantageous use of visible light [11].

4. Conclusions

Titania was used as the base catalyst material of choice for solar hydrogen production, due to its stability and non-corrosive properties, as well as environmentally friendliness and low cost. The need to decrease the electron-hole recombination rate was accounted for by platinum deposition and the addition of ethanol as a hole trap.

o Gas production rates were found to be linear with time at near room temperature.

o pH variations during hydrogen production were striking changing from 11 to values as low as 5.6, this is thought to be due to the formation of acetic acid during the reaction, accounting for the lower concentration of CO2 and CH4 found by gas chromatography Keeping the pH in the alkaline range ensured a constant rate of gas production for extended periods of time.

Further work is needed to identify low cost metal loading materials with acceptable enhancement for hydrogen production, as well as modified catalysts that allow effective utilization of visible light.

Acknowlegments

Acknowlegments are due to B. Charasse, J. Chesnau and M. Pinho for assistance in some of the experiments.

References

[1] A. Fujishima, A Honda, Nature, 238 (1972), 37-38.

[2] — Y. Z. Yang, C. H. Chang, H. Idriss, Appl. Catal. B, 67 (2006) 217-222.

[3] — G. R. Bamwenda, S. Tsubota, T. Nakamura, M. Haruta, J. Photochem. Photobiol. A, 89 (1995) 177-189.

[4] — A. R. Gandhe, J. B. Fernandes, Bull. Catalysis Society of India, 4 (2005) 131-134.

[5] — S. Pilkenton, Son-Jong Hwang, D. Raftery, J. Phys. Chem. B, 103 (1999) 11152-11160.

[6] — Z. Zaina, L. K. Hui, M. Z. Hussein, Y. H. Taufiq-Yap, A. H. Abdullah, I. Ramli, J. Hazardous Materials, B125 (2005) 113-120.

[7] — J. C. Kennedy III, A. K. Daty, J. Catalysis, 179 (1998) 357-389.

[8] — G. Marci, V. Augugliaro, A. B. Prevot, C. Baiocchi, E. Garcia-Lopez, V. Loddo, L. Palmisano, E. Paramauro, M. Schiavello, Societa Chimica Italiana, Annalli di Chimica, 93 (2003) 693-644.

[9] — C. G. Silva, W. Wang, J. L. Faria, J. Photochem. Photobiol. A, 181 (2006) 314-324.

[10] — R. Gouveia, R. A. Silva, C M. Rangel, Mat. Sci. Forum, 514-516 (2006) 1385-1390.

[11] — Y Li, C. Xie, D. Peng, G. Lu, S. Li, J. Mol. Catal.: A Chem., 282 (2008)117-123.

The solar dryer presented is an alternative for drying woodchip in Scotland. Dryer and solar collector are parts of the solar dryer and have been presented and analysed separately. Woodchip drying performance has been described for certain conditions in a range of low temperatures, relative humidity and low drying velocities. Concluding that increments in either flow rate or drying temperature reduces the drying times. Page model has been found to be a simple and accurate model that describes with good agreement the moisture content curves as a function of drying velocity and temperature.

The solar thermal system presented is a particular prototype based on the transpired plate collector. The connection between PV-panel and fan comes to a low threshold irradiance that allows the system works in poor light conditions. The thermal system increases considerably the ambient temperature in all the range of irradiance and delivers flow at high temperatures with high efficiency at high irradiance levels.

In future work, in order to optimize the solar collector performance, the configuration of the collector may be modify: hole distribution of the absorber, material employed, the using of glazing cover. Once dryer and thermal systems are completely assessed, it will be possible to draw main conclusions about using solar energy for drying woodchip in Scotland.

References

1. FREDS, Forum for Renewable Energy Development in Scotland. Promoting and Accelerating the Market Penetration of Biomass Technology in Scotland, B. E. Group, Editor. 2005: Scotland.

2. Commission, S. D., Wood Fuel for Warmth. June 2005, Scottish Executive: Scotland.

3. Timo Tahvanainen, A. A., Storing of energy wood and wood fuel quality management, F. (Metla), Editor. 10 11 2006: Soria. Finland.

4. Healion, K., Wood as a Renewable Source of Energy. 2002, COFORD Connects, Socio-Economic Aspects of Forestry.

5. Odeh, N., et al., Modelling of flow rate in a photovoltaic-driven roof slate-based solar ventilation air preheating system. Energy Conversion and Management, 2006. 47(7-8): p. 909-925.

6. Grassie, T., Modelling the outlet temperature of a PV driven transpired solar air heater, in SOLARIS New Delhi, India.

7. Brenndorfer, B., Solar Dryers: Their Role in Post-harvest Processing. 1985: Commonwealth Secretariat.

8. Midilli, A. and H. Kucuk, Mathematical modeling of thin layer drying of pistachio by using solar energy. Energy Conversion and Management, 2003. 44(7): p. 1111-1122.

9. Ekechukwu, O. V., Review of solar-energy drying systems I: an overview of drying principles and theory. Energy Conversion and Management, 1999. 40(6): p. 593-613.

10.Sharma, G. P., R. C. Verma, and P. Pathare, Mathematical modeling of infrared radiation thin layer drying of onion slices. Journal of Food Engineering, 2005. 71(3): p. 282-286.

11. Duffie, J. A. and W. A. Beckman, Solar Engineering of Thermal Processes. 1991: Wiley-IEEE.

S. Alexopoulos1*, J. Gottsche1, B. Hoffschmidt1, C. Rau1, P. Schwarzbozl2

1 Aachen University of Applied Sciences (AcUAS)/ Solar-Institut Julich (SIJ); Heinrich-Mufimann-Strafie 5;

D-52428 Julich

2 German Aerospace Center (DLR), Institute of Technical Thermodynamics, Linder Hohe, Porz-Wahnheide,

D-51147 Koln

* Corresponding Author, alexopoulos@sij. fh-aachen. de

Abstract

Solar thermal power is an emerging technology that provides clean electricity for the growing energy market. To the group of solar thermal power systems belongs the solar tower where a field of two-axis tracking heliostats reflect the solar radiation to the receiver aperture, where concentration ratios up to 1000 are reached. This offers the potential to provide high steam temperatures for a rankine cycle or ultimately even high enough temperature heat for the gas turbine system of a combined cycle power plant.

One major option for the accelerated market introduction of solar thermal power technology is the concept of solar-fossil hybrid power plants. Their advantage, compared to solar-only systems, lies in low additional investment costs due to an adaptable solar share and reduced technical and economical risks.

In this paper a simulation model for the calculation of a hybrid power plant is described. Also the design and performance assessment of solar hybrid tower plants in the power level of 1-2 MW, is presented. An advanced software tool library is developed for the modelling of such small hybrid power plants. First validation and simulation results for parts of a small solar tower power plant are described and discussed.

Keywords: solar thermal power, central receiver system, solar tower, hybridization, simulation

1. Introduction

Solar tower power plants are an innovative type of power production facility based on solar radiation as primary energy resource instead of conventional combustion processes. A 1.5 MWel demonstration plant with volumetric air receiver is being built in Juelich, Germany ([5], [7]).

In the primary cycle of the Juelich concept, air is heated up to about 700°C and used to generate steam in a heat recovery steam generator (HRSG) (see Fig.1 without consideration of the burner). Steam parameters in the secondary cycle may be up to 30 bar and 500°C. The steam drives a turbine/generator system and returns in form of condensate to the steam generator. A thermal storage system is connected parallel to the boiler. When charging, hot air passes through the vessel filled with ceramic material which develops a temperature profile between the hot and the cold end of the storage. The cold air exiting the steam generator and/or storage is returned to the receiver. To discharge the

storage, cold air from the steam generator is circulated in reverse flow through the storage vessel and back to the steam generator. The air, which delivers almost all its energy in the heat transfer process, leaves the boiler component with a temperature of about 120°C. After the boiler, a blower forces the air to mix with the portion of air that has delivered its energy to the thermal storage. A second blower located after the thermal storage forces the total combined flow of air to the receiver [1].

This system concept offers several benefits. Air as heat transfer medium is available for free, non-toxic and does not require freeze protection during times of non-operation. Operation with steam parameters as customary in conventional power plant engineering ensures high efficiency and optimum use of the available solar energy. Major parts of the plant can be built by using standard components from conventional power plant construction.

In Juelich (North Rhine-Westphalia, Germany) the first solar tower plant with gas as heat transfer medium is under construction. The concept of the solar tower power plant Juelich (STJ) is the result of the development of the solar power plant technology with an open volumetric receiver. An open volumetric receiver enables the better exchange of energy between the inserting solar power and the transport medium. The incident radiation is absorbed from the receiver modules and is connected by convective heat transfer with the flow of ambient air which is moved trough the structure [2]. The STJ will finish construction by the end of 2008 and begin feeding electricity into the public grid for the first time.

In order to enable the plant to produce electricity in periods where the solar radiation is not available a hybridization concept is been taken into consideration. The concepts of hybridization consist of the use of a gas burner or a gas turbine as an auxiliary system.

This is an important step towards cost reduction of solar thermal power. The advantages of hybrid power plants are a variable solar share, a fully dispatchable power and a 24 h operation without storage.

The hybridization of a solar thermal power plant provides an increase of the efficiency and availability. In regions with very high radiation, solar thermal power plants with heat storage facilities can reach a maximum of 3.000 to 4.000 nominal load hours per year. Through hybridization, e. g. by combustion of biofuels, the production of electricity can be increased to almost 8.600 hours. It is expected that such hybrid power plants will have a high potential for market introduction in the next decade.

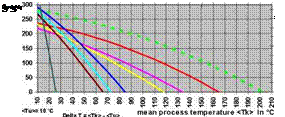The thermal efficiency factor is also known as collector efficiency factor. It can be calculated easily with the help of standardized parameters, e. g. from the Solar-Keymark-Tests, for every collector at any operating point. The characteristic curves in the following diagram are converted concerning gross collector area because this is really relevant. Corresponding to this area money is paid, subsidies are granted, parameters are compared and installations are planned.

fig. 9 — Heat power performance of collectors at an irradiation of 400 W/m2

The temperature range started at <TU> = 10 °C, a typical German average outdoor temperature. So with the displayed process temperature also the mean collector temperature and except for 10 K initial displacement also AT is shown. The diagram demonstrates that with temperatures between 35 °C and 50 °C all flat plate collectors drop under 150 W/m2 whereas this is not the case with all tube collectors before temperatures between 60 °C and 140 °C are reached. The green broken line represents a „theoretical“ CPC vacuum tube collector that could be manufactured according to the state of the art.

If there is no temperature difference AT, every collector has an individual maximal efficiency factor p0. The higher AT, i. e. the hotter the collector is, the smaller is the efficiency factor. The bigger the heat losses are, the sooner the efficiency factor reaches zero with rising collector temperatures. However, the efficiency factor decreases faster with weak than with strong irradiation Gs. Thus collector sellers try to present the efficiency factor with high irradiation, e. g. with 700 W/m2, 800 W/m2 or sometimes even with 1000 W/m2. But this is unrealistic in Central Europe. Wurzburg gets only 11 % of the irradiation with 800 W/m2 or more, but about 13 % with less than 200 W/m2 (fig. 10). The yearly average of 397 W/m2 ignores the range below 100 W/m2 because it is completely irrelevant for flat plate collectors and brings only about 5 % of the yearly energy harvest for tube collectors. Otherwise the average would be even lower. In the following we calculate with 400 W/m2.

The thermal efficiency factor says how efficient the heat is gained from solar energy but says nothing about the part that could be used e. g. in a heat engine. This part is expressed by the Carnot factor i)C (fig. 11). nc for a collector is:

<TK> mean collector or process temperature

<TU> mean ambient outdoor temperature with <TU> =

273 K distance between Celsius scale and absolute zero

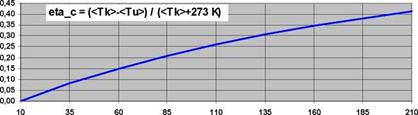fig. 11The Carnot
factor nC

nc says how much of an amount of heat could be transferred into power or electricity. It is about 40 % with 200 °C process temperature. This consideration is interesting because you could think of solar heating systems that transfer surplus heat into electricity and feed it into the network. Efficiency factors as known from photovoltaics are realistic.

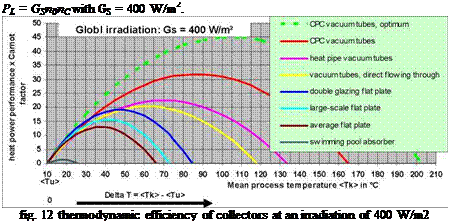The thermal collector efficiency factor multiplied by the Carnot factor and the total irradiation Gs results in the collector performance value depending on the process temperature <TK>. The performance value weights the achievable heat performance of a collector concerning the universal thermodynamic usability.

All performance value characteristics start at zero. Heat at the level of the mean ambient temperature is useless. Collectors with high p0 have the steepest slope. Collectors with the flattest thermal characteristics have the longest slope. Sometime the decrease of the collector efficiency factor will outweigh the increase of the thermodynamic efficiency factor with every collector. At the characteristic collector temperature TC you get a maximum. Then the performance value decreases and reaches zero exactly together with the thermal efficiency factor because the thermal gain decreases with higher temperatures more and more.

A collector reaches its performance maximum at the characteristic collector temperature TC. TC changes analogous to the irradiation and can be calculated for every collector from the data of the Solar-Keymark-Test. Every collector can be used at lower temperatures than TC. But it makes no sense to use collectors at much higher temperatures than their specific TC (e. g. flat plate collectors for solar cooling or for heating support in condominiums where in every season the temperature must exceed 65 °C because of Legionella bacteria).

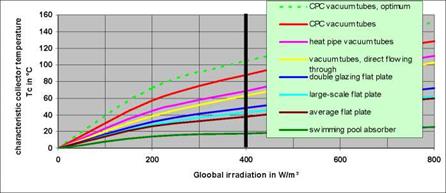The characteristic collector temperature depends on the location. The black line at 400 W/m2 represents Wurzburg. Mean irradiation up to 600 W/m2 is reachable (possible) in the Mediterranean area, up to 650 W/m2 in California, up to 700 W/m2 in Australia and India and even up to 750 W/m2 in the Middle East.