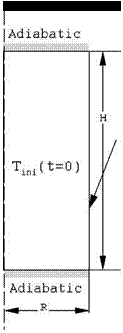
The computational domain includes the fluid inside the tank and the metallic wall. For the energy equation, it has been set that heat transfer to the ambient is dominated by convection, by imposing an a cosntant temperature at the sidewall while the top and the bottom have been considered adiabatic. At the symmetry line (r=0,z), gradient of temperature in radial direction has been assumed null. For the momentum equations, non-slip conditions at every solid wall, have been imposed. At the symmetry line, radial velocity has been set to zero, while the gradient of axial velocity component in radial direction has also been assumed null.

1.2. Discretization method

The numerical solution has been obtained by discretization, in finite volumes method and with fully implicit temporal differentiation, using cylindrical staggered grids as described by Patankar

[9] . Diffusive terms have been evaluated using a second order central differences scheme, while convective terms have been approximated by means of a SMART [10] scheme using a deferred correction approach. Pressure-velocity coupling was obtained by SIMPLEC method [11]. The resulting algebraic system of linear equations have been solved using the TDMA [9]. The criteria to stop transient simulation has been set as a function of the non-dimensional mean fluid temperature. As an infinite time is needed to fully cool down the fluid in the cylinder (that is, to reach exactly вл(т) = -1), it is necessary to terminate the numerical simulations at some point. In

this study, the criteria to stop the transient simulation has been taken as a general rule, all numerical simulationsn will be terminated when t = Tf, that is when QJj) = -0.99, the iterative

procedure has been truncated once the non-dimensional variables increments and residuals are lower than 10-5.

Because of the large variation near to the interface solid-liquid, it was necessary to use a mesh concentrates points in the boundary layer and is relatively coarse in the interior [12].

Fig. 1. Schematic of the physical system and the computational domain.